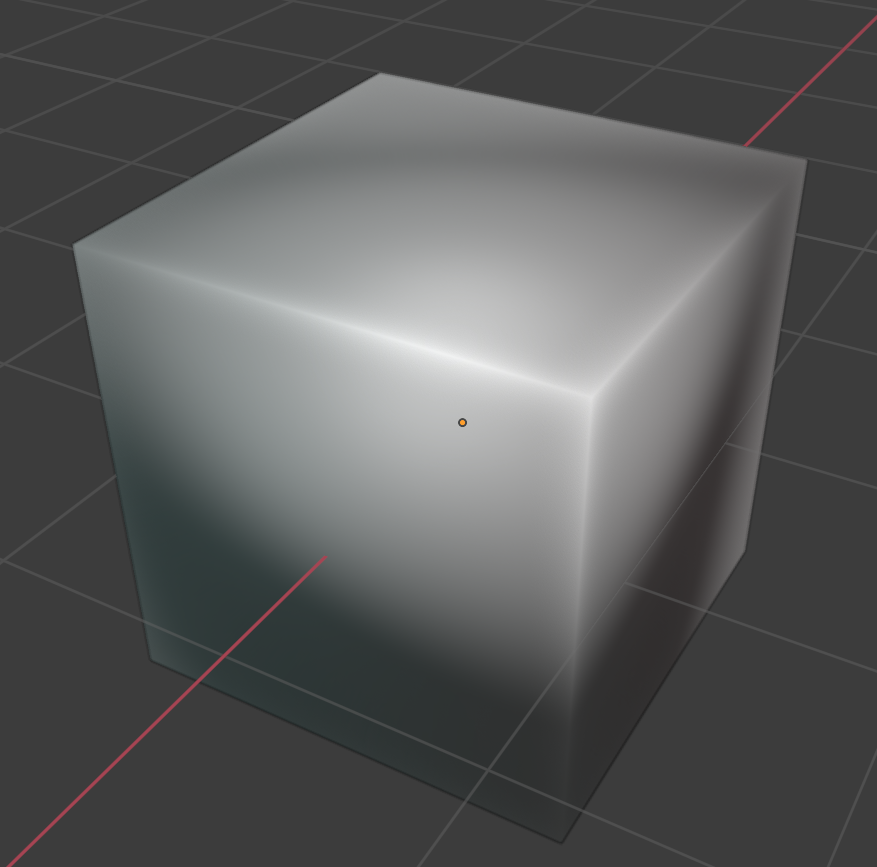
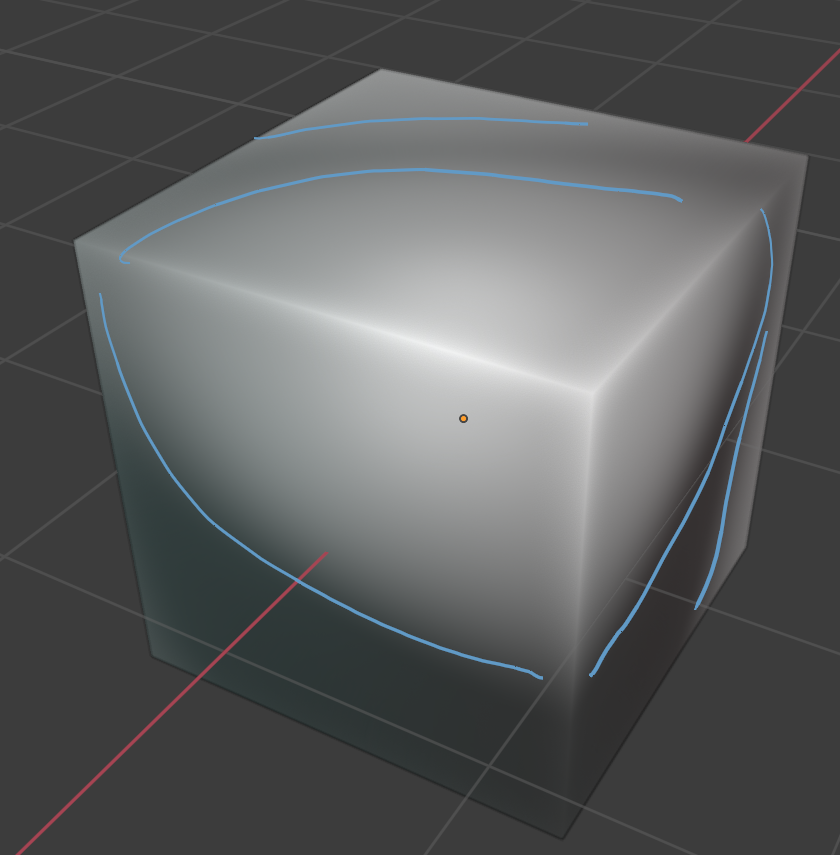
The normals at the vertices are indeed averaged, so they point directly away from the origin, just like a sphere. So you can think of the cube as a really low-poly approximation of a sphere.

When a light hits a true sphere, it divides it into two hemispheres, a "bright" side that faces the light, and a "dark" side that faces away from the light. The bright side is brightest near the light and fades into shadow as you move away from it. The dark side receives no light so it is totally dark everywhere.
The dividing line between the two sides is a circle, the "equator" that cuts the sphere into the two hemispheres.

However on a triangle (all meshes are made of tris), the dividing line will instead be approximately a straight line. (The reason is basically that the normal is (approximately) linear in the location on the tri, and the equation for the dividing line (N dot L = 0) is linear in the normal, which means the solution set is a straight line on the tri.)
So on a low-poly sphere the diving line between light and dark looks like a low-poly "circle". There is one straight line segment on each face.
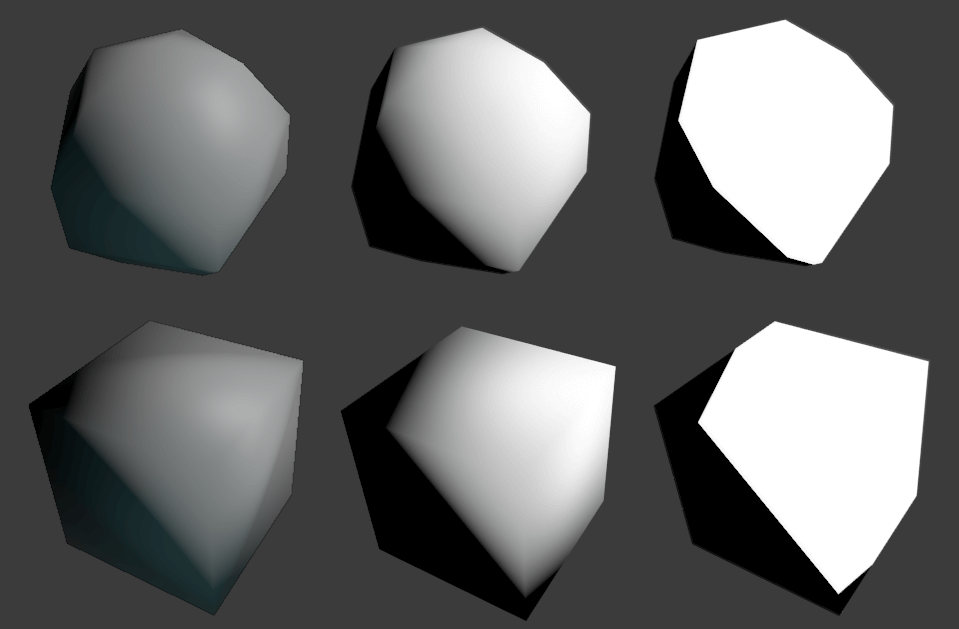
I hope that explains some of the shape you see.