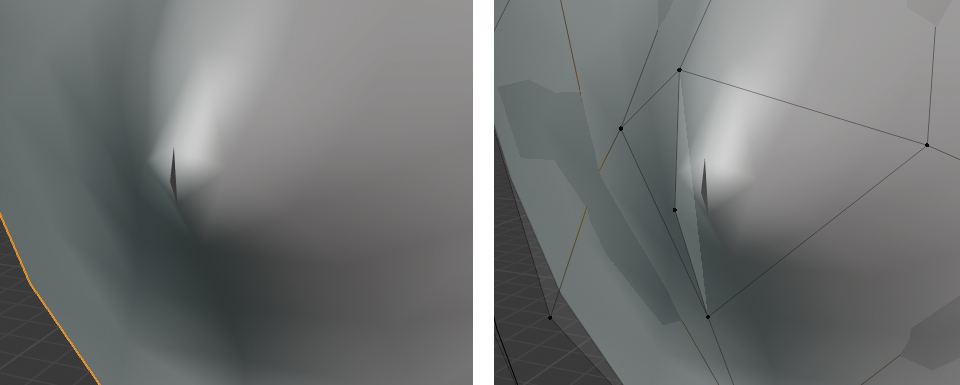
Catmull-Clark subdivision works well-- meaning, it makes smooth meshes-- under certain circumstances. One of those circumstances is roughly planar quads. Here, your quads are most definitely not-planar. How can we tell? The biggest clue is that you have two quads that share three vertices. That should never happen; adjoining quads should only ever share two vertices (ideally, for a 3- or 4-pole) or one vertex (for a 5-pole). You have a pair of strongly concave faces.
We can fix it just by insetting a new face in those two, then scaling that new face down in it's normal Z axis to make it more planar:
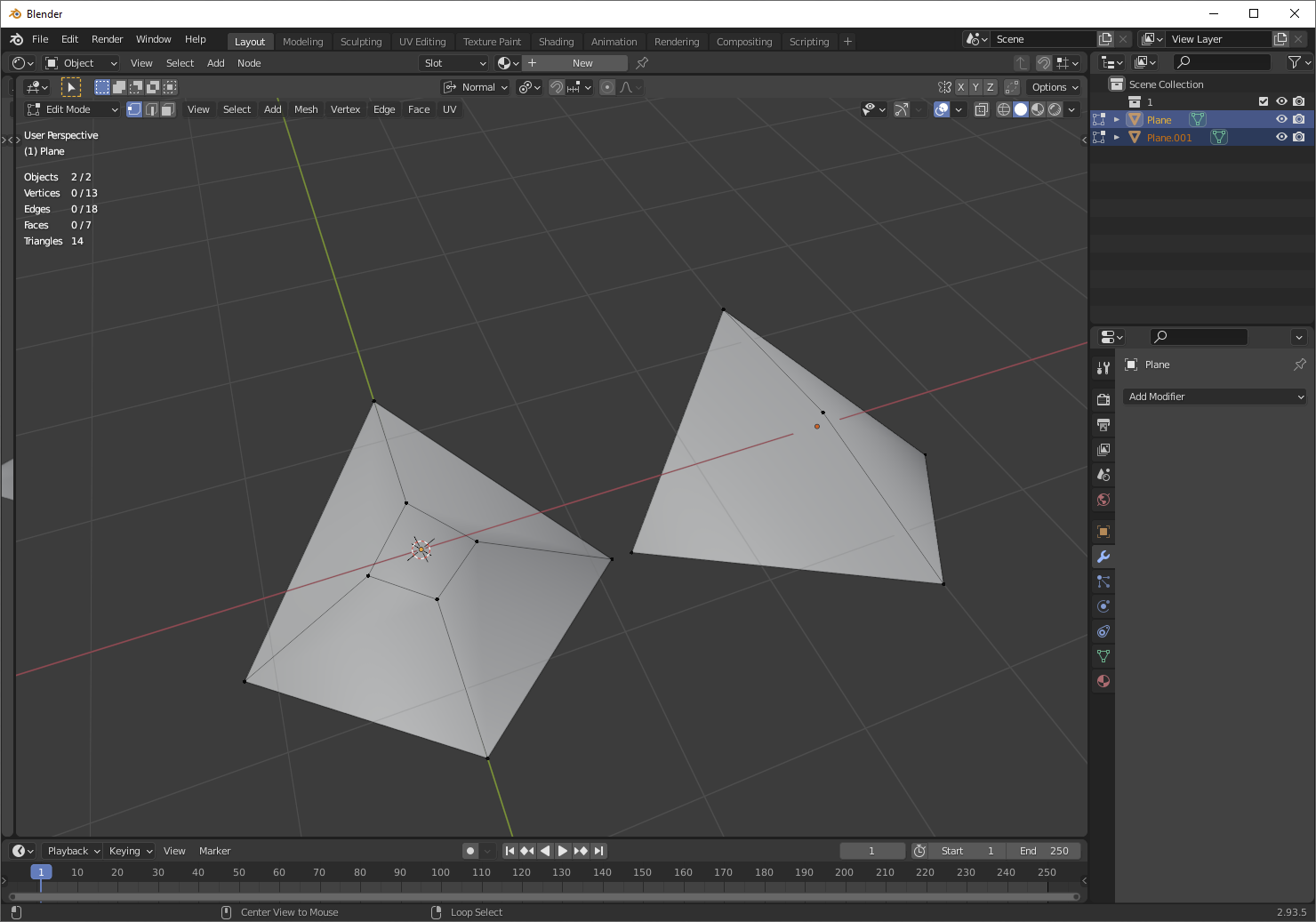
This does come at the cost of creating non-planar 3-poles, which are to be avoided for C-C subdivision, but are not as serious of a problem.
But also, poles can be moved to better locations; the more planar the neighborhood of the pole, the better. Techniques for moving poles are dealt with elsewhere so I won't cover them in this answer.
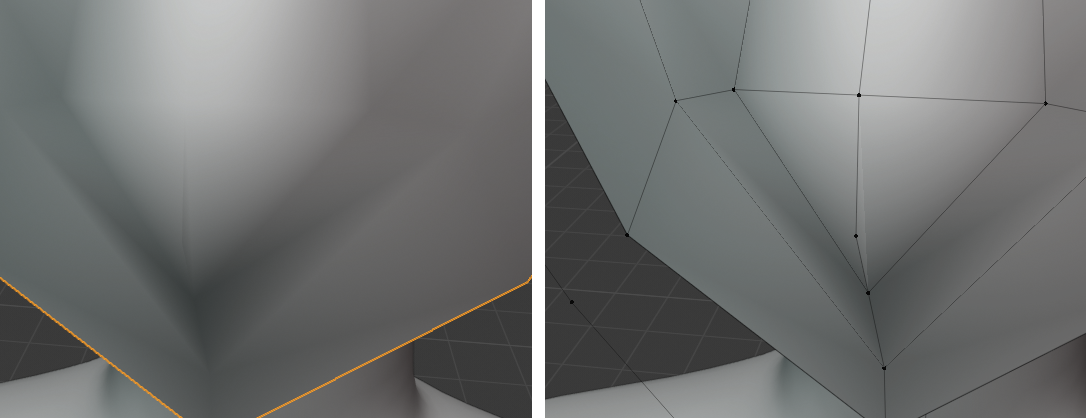 1 subdivisions:
1 subdivisions: