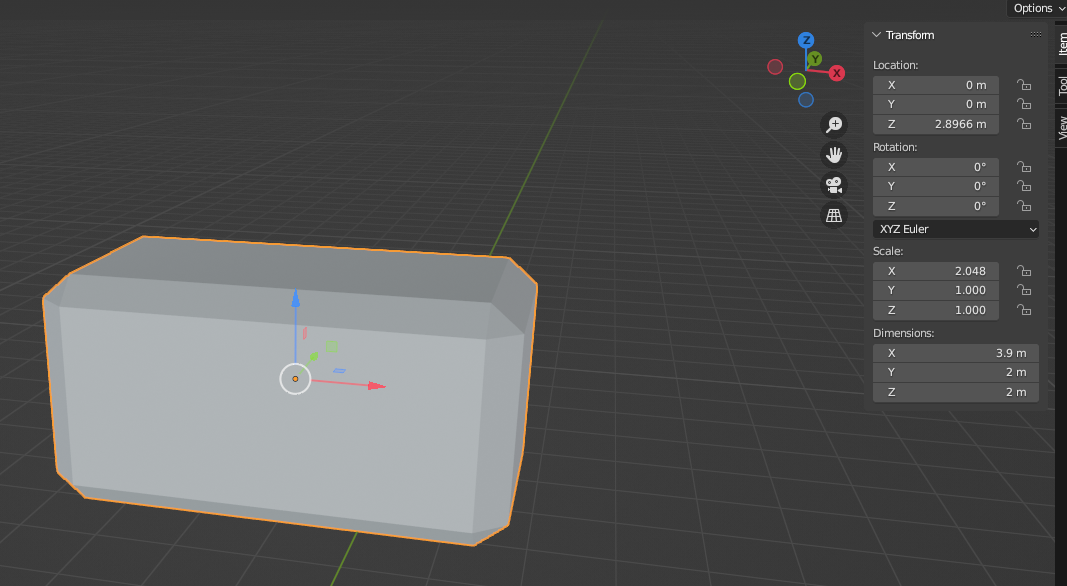
So I accidentally applied the rotation of an arm and its to late to undo. Is there a way to fix this accurately?
$\begingroup$
$\endgroup$
3
-
$\begingroup$ There's no way to "fix" that. You have possibility to use Normal transform orientation, create custom transform orientation or align view with face and then transform according to View transform orientation. $\endgroup$– Mr ZakCommented Oct 18, 2016 at 19:21
-
$\begingroup$ maybe you can align/rotate another simple object to the arm, then use a "copy rotation" constraint on the arm to copy its rotation, but with "invert", then use "apply > visual transforms"... then apply rotation... I tried on a simple shape... it depends on your specific case though... $\endgroup$– m.arditoCommented Oct 18, 2016 at 20:52
-
$\begingroup$ Also rotating the vertices by snapping active vertex to a reference point can yield good results although it will only be 99,999% perfect. Afterwards you can try to find a small enough zoom level in ortho view so you can snap the vertices to grid. $\endgroup$– kheetorCommented Oct 19, 2016 at 6:47
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
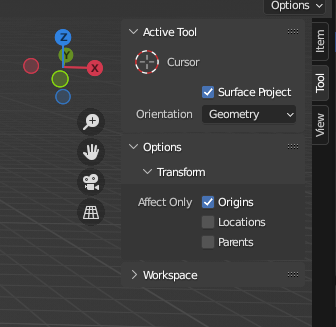
This can be done by using the "Geometry" orientation with the cursor tool,
and the "Affect Only Origins" option selected.
Here's an object with its rotation applied, click on the face you want reset to the correct position with the cursor tool, in this case it would be the top face.
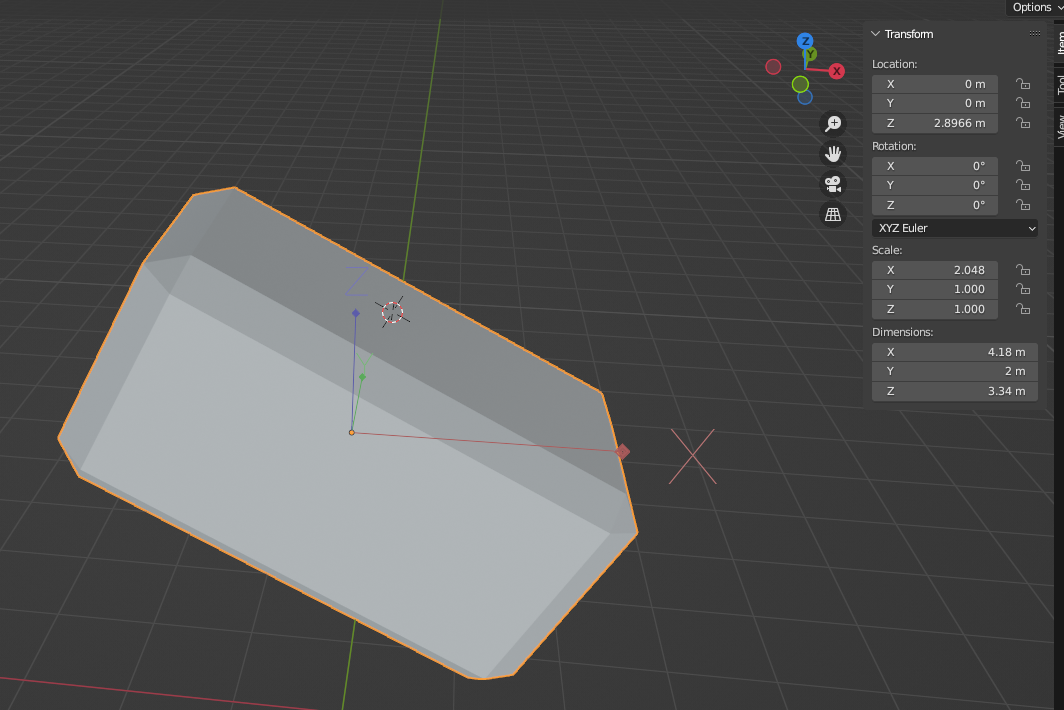
Now, Shift-S > Cursor to Selected (make sure the object you want to reset is selected) - This will make sure the position transformations stay the same and only rotation origins are changed
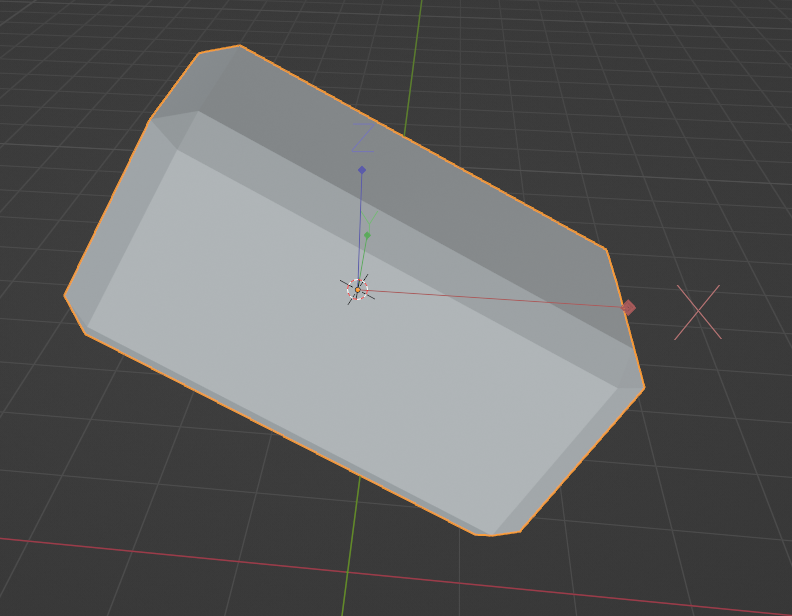
Finally, make sure your transform orientation is set to "Cursor" and go to Object > Transform > Align to Transform Orientation
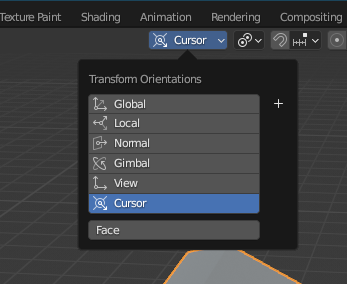
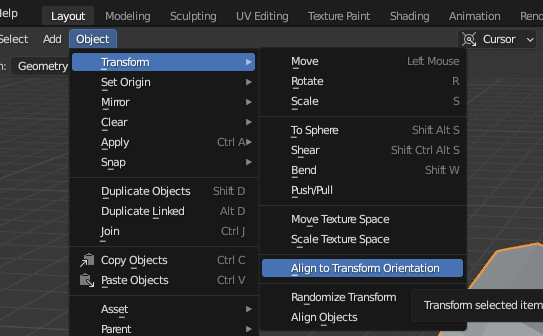
You should now see that your applied rotation has been fixed, and if you set the rotate values back to 0 the object will be pointed perfectly up. Don't forget to disable "Affect Only Origins" so you are able to transform your object again.
