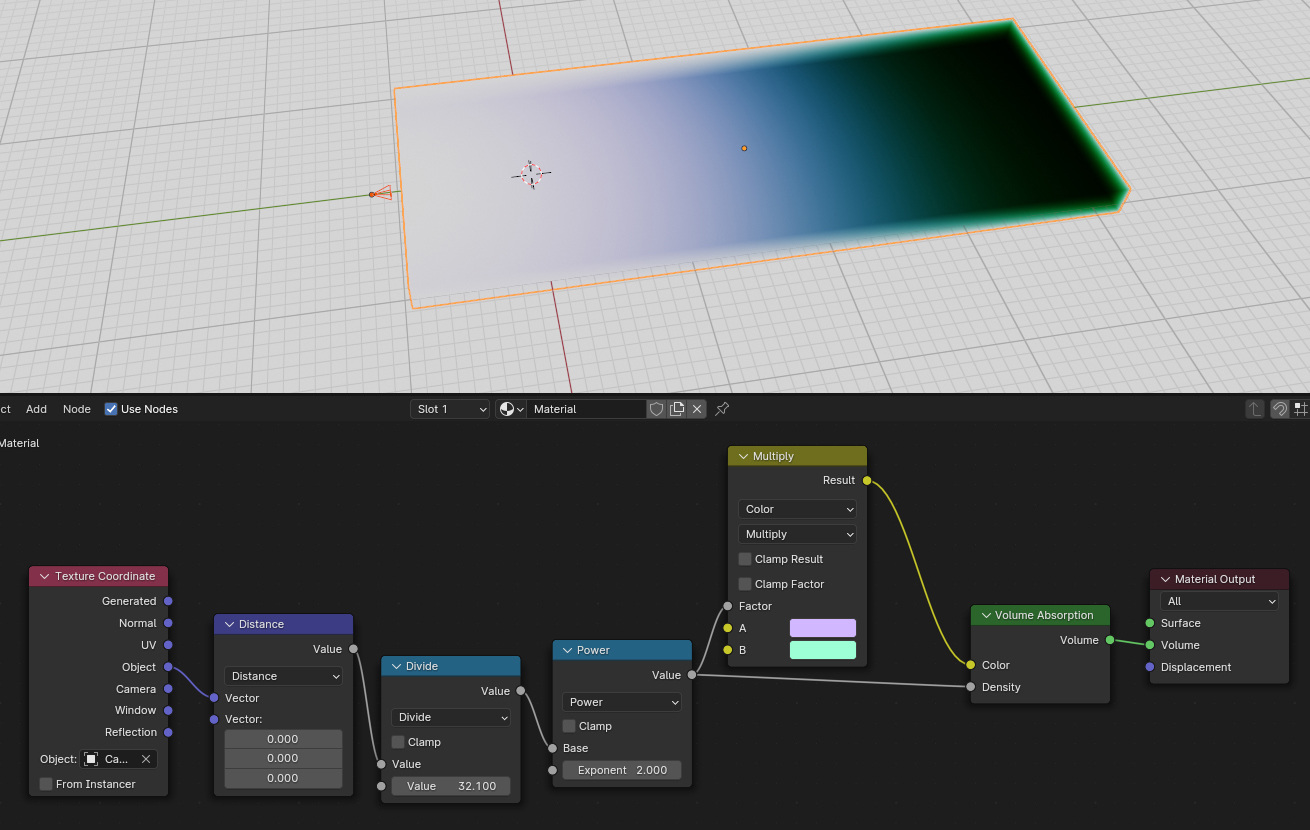
What you did not tell us is what the base color of the absorption should be for the R, G and B channels which you want to multiply with $z$ and different factors? I suspect you are starting off with R = G = B, so some greyscale value. Let's call this value $x$. The result you want is $(zx, 3zx, 2zx)$ or
$$z(x, 3x, 2x).$$
Now choose any arbitrary value for $x$ (preferably one that is within the 0 to 1 range when multiplied by 3), for example $\frac{1}{3}$. Place it in the term above instead of $x$ and you get
$$z(\tfrac{1}{3},1,\tfrac{2}{3}).$$
So you just give the Volume Absorption node a color of (0.333, 1, 0.667) and plug the distance $z$ in the Density like before. To change the density overall without changing the realtions of the colors to each, you can multiply or divide $z$ with some value.
That is the simple version - but that's basically just giving the Volume Absorption a fixed color. Although this is what you are asking for, I'm not sure if you thought of it to be intensifying the three colors differently from each other.
To do this and have a visible effect it would be better to start off with some different color and multiplying this with the $(x, 3x, 2x)$ greenish color using the distance as mix factor, like the example below: there I start off with some violet absorption and the green intensifies with distance from camera.