0
$\begingroup$

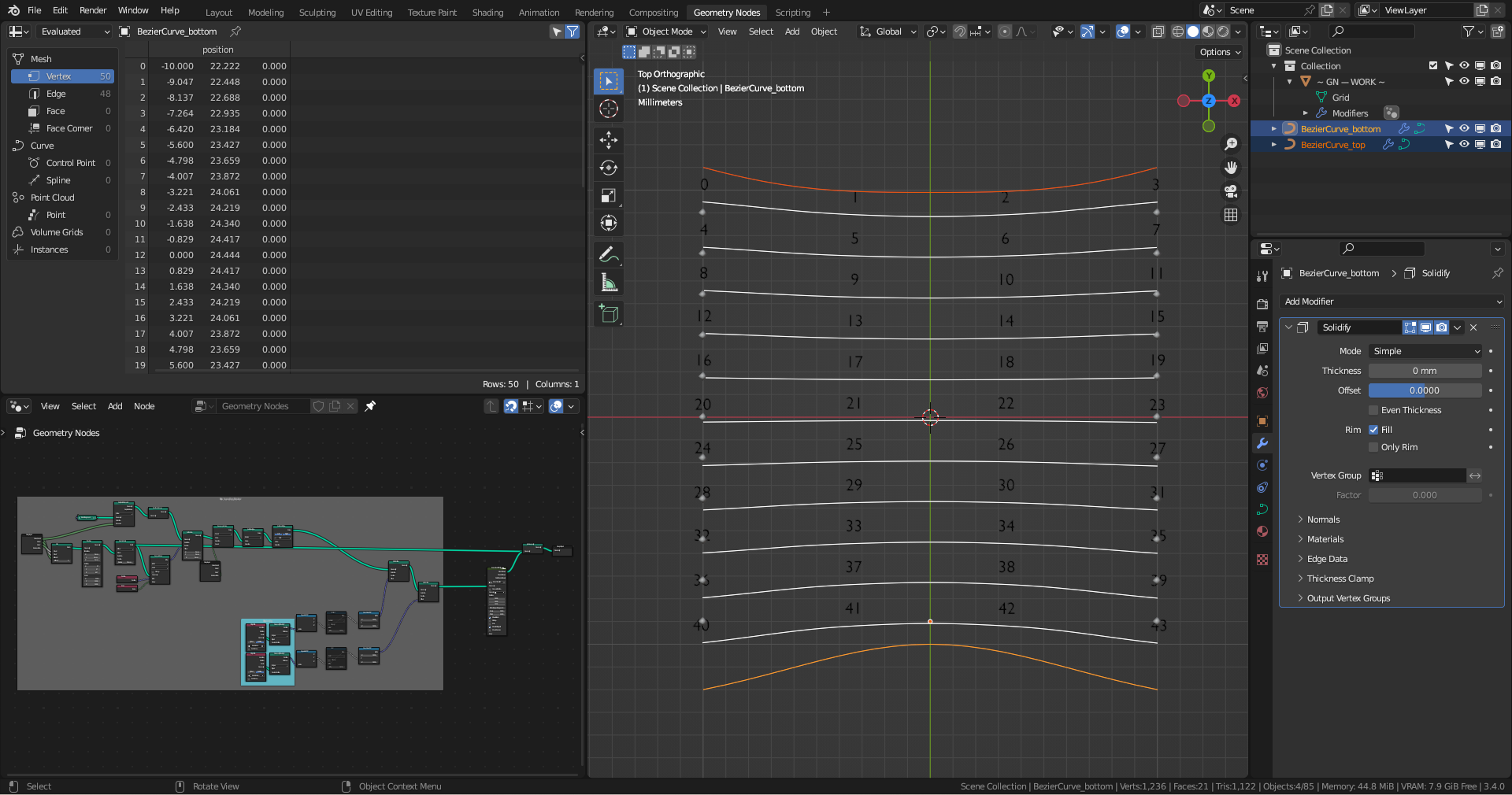
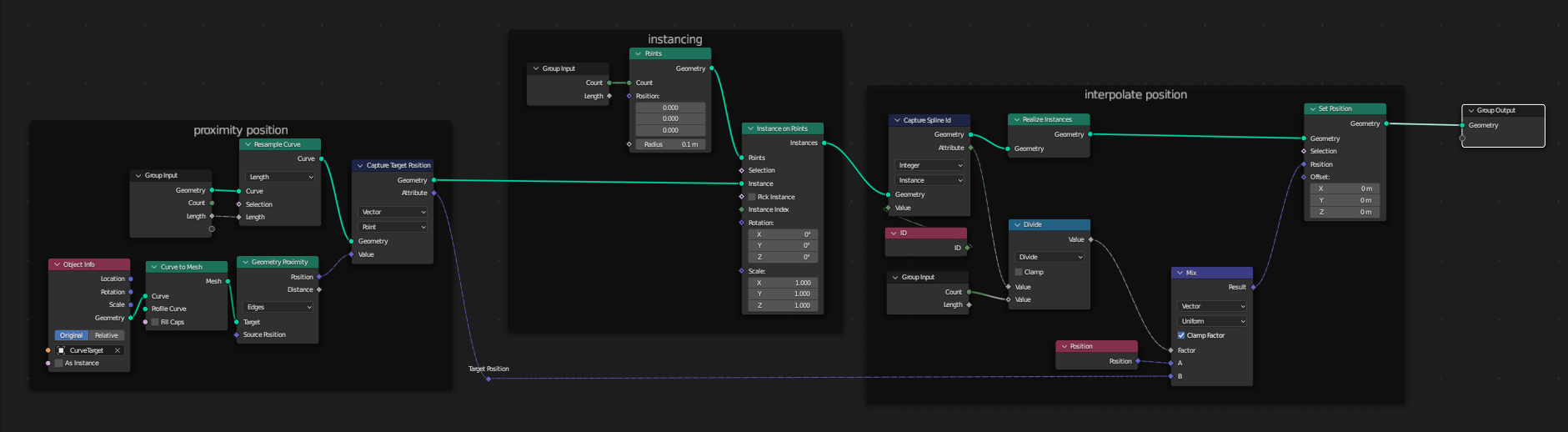
since my last post GN: How to adjust Bezier-curves with geometry proximity to get streamlines? I've managed to get the streamlines into dependency of the two outer reference curves.

enter image description here

Now after numerous attempts I am still facing one or the other problem:

  • The streamlines now follow the curvature of the two reference curves and blend / distribute into each other from top to bottom. For this I plugged the output of the Geometry Proximity node into the offset input of the Set Position node. Unfortunately, the start and end points of the streamlines also shift. How can I adopt the respective curvature, but keep the start and end point of the streamlines in the old position or transfer them back? 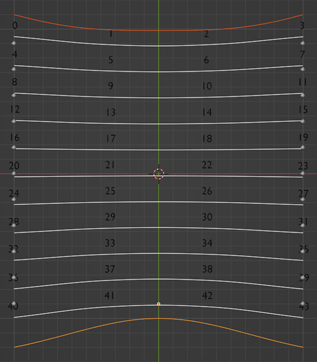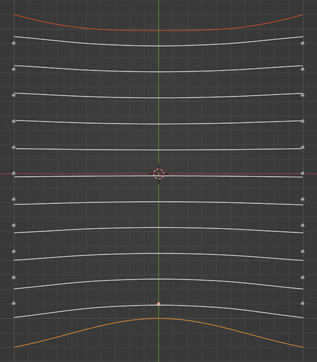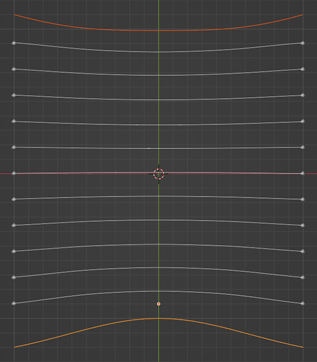enter image description here

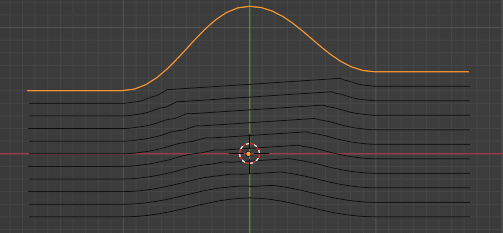
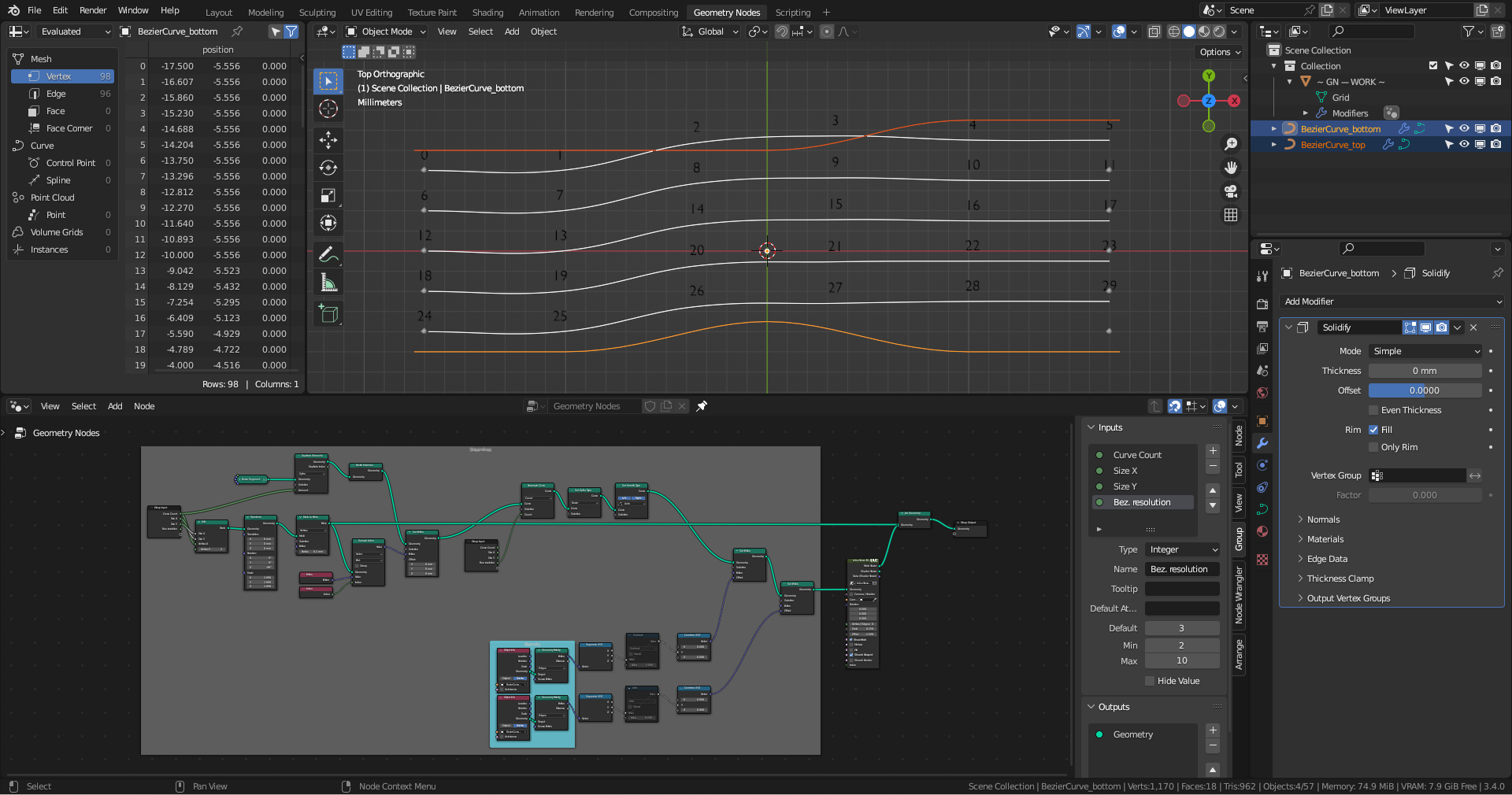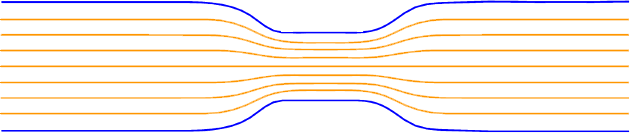
  • As the reference curves get more complex, the streamlines no longer follow them exactly, even if I increase the resolution of the streamlines using the resample curve node. enter image description here How can I control the influence of the Geometry Proximity node? The Map Range Node didn't work for me or I wasn't able to use it properly. The goal is still to achieve this: enter image description here

I hope someone can help me to find a solution. I'm also very grateful for every tip!

Have a nice remaining weekend everyone!

Improve this question
$\endgroup$

1 Answer 1

Reset to default
3
$\begingroup$

3d Setup

Let's use only two curves. A source curve and a target curve. We are going to add geo nodes to the source curve, to instance it and the interpolate the duplicated splines to the proximity position.

  1. Resample the curve and get the closest position of the target curve (with the Proximity Node). Store the target position with the Attribute Capture Node.
  2. Instance the curve a few times in place. Capture the instance id and then realize the instances.
  3. By calculating captured_id / instance_count we will have a relative value [0, 1) to mix the original point position with the target position.
  4. Use the captured target position to mix the positions.

node setup

This setup is limited by the geometry proximity, but that was the premise of your questions title.
proximity problems

Improve this answer
$\endgroup$
2
  • $\begingroup$ That's exactly what I was looking for!! Thank you @Leander 😊 $\endgroup$
    – pb1893
    Commented Mar 5, 2023 at 18:50
  • $\begingroup$ @pb1893 If your question could be answered satisfactorily, please consider marking it as "Accepted Answer" so that this question appears as solved. Thank you! $\endgroup$
    – quellenform ♦
    Commented Mar 10, 2023 at 21:35

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .