1
$\begingroup$

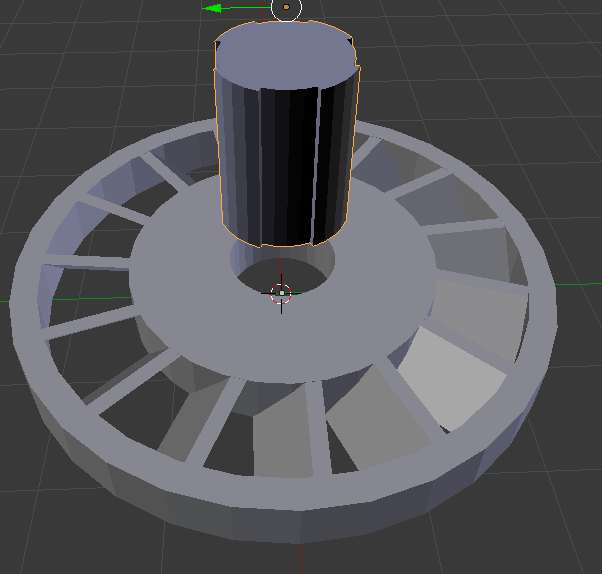
I want to subtract the upper cylinder from object below. enter image description here

Using the Boolean modifier in difference-mode, the complete object disappears.

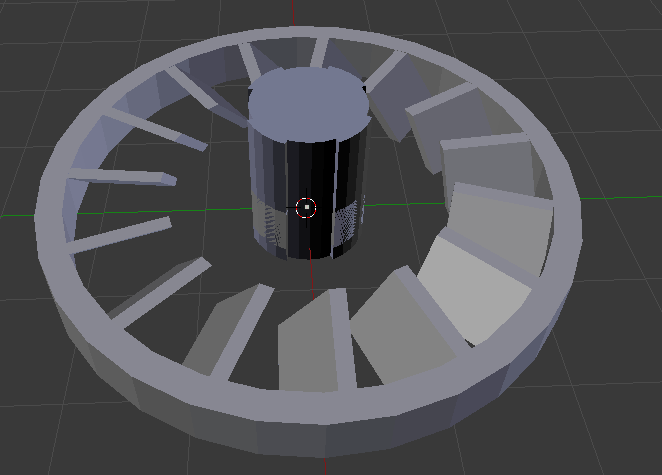enter image description here Here is the Blender-File

PS. I am new and almost always objects react weird when i am following a tutorial (like Array around an object).

Improve this question
$\endgroup$
2
  • 3
    $\begingroup$ A comprehensive post on booleans can be found here: Boolean Modifier not working $\endgroup$
    – user1853
    Commented Mar 7, 2018 at 20:22
  • $\begingroup$ Remove Boolean modifier from the wheel. Select the vertical cylinder and add Boolean to this object, in the Boolean Modifier window select "Object: InnererK", then "Operation: Intersect". This will do what you want I think $\endgroup$
    – MicroMachine
    Commented Mar 7, 2018 at 23:14

2 Answers 2

Reset to default
0
$\begingroup$

It looks like you need to fix/flip/recalculate your normals on the top cylinder. To fix it...

Select it

Tab into edit mode.

T to toggle the tool panel @ left.

Click the Shading/UV tab.

Click Flip Direction

Tab to toggle back to Object mode.

Ctrl + A >> Rotation & Scale.

Try your boolean operation again, make sure the difference option is set.

Improve this answer
$\endgroup$
2
  • $\begingroup$ I don't know what that is doing (I will look into it), but it works. Thank you. $\endgroup$
    – DerBauerimBenziner
    Commented Mar 7, 2018 at 22:08
  • $\begingroup$ When I answered this, I was really trying to find an answer on blender.stackexchange.com that I remembered seeing a while back ago that fit your case, but for the life of me I couldn't; but then I found the post Here it is. It should explain your issue fairly clearly. $\endgroup$
    – Rick Riggs
    Commented Mar 8, 2018 at 2:41
0
$\begingroup$

You might also try another couple of things if flipping the normals doesn't get it done. I find that nine out of ten times a boolean doesn't work because of scale rotation issues. cntrl>A on each object in the scene individually to make sure that the rotation and scale are all the same. I also find the bool tools addon absolutely brilliant.

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .