1
$\begingroup$

Clone trooper mesh I downloaded from the internet

NOTE: I'm new to Blender/3D modeling, and this is my first project.

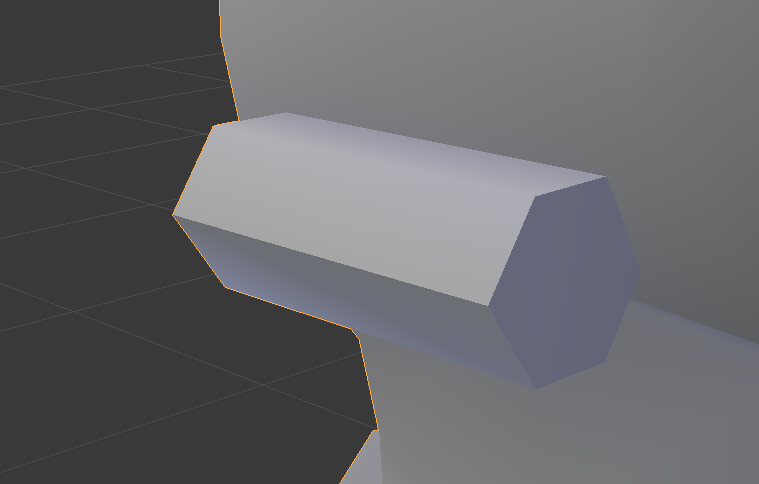
Here is a mesh of a clone trooper from a game from 2005 that I'm trying to "modernize." This hexagonal prism on his back is supposed to be more of a cylinder, but due to it's low-poly nature, it's obviously not. I've tried subdividing and smoothing the rectangular faces, but it bows the cylinder out in the middle as well as messing up the 90 degree angles between the rectangular faces and the hexagonal faces (possibly in an attempt to smooth those angles? which I don't want).

What's the best way to achieve a more cylindrical shape without having to manually bisect each face and translate the sides diagonally to make it more round? (If this is the only way, how can I perfectly translate the sides on the X and Z-axis EQUALLY so that I don't have to eyeball it?)

Improve this question
$\endgroup$

1 Answer 1

Reset to default
2
$\begingroup$

In edit mode, use Ctrl-R to add loop cuts to the sides of the 6 sided cylinder. Once you have an even division, select the vertices of the cap or end hexagon. Using Loop Tools addon, choose LoopTools> Circle, and the new vertices will form a circle. Repeat for the other hexagon. edit: Here is how I would approach it. 1. Original 2. Tris to quads 3. Delete the vert in center of the hexagon 4. ctrl-R loop cut the faces 5. LoopTools Circle to get the round 6. Extrude and carefully fill with quads 6 step image

Improve this answer
$\endgroup$
3
  • $\begingroup$ That didn't work because the rectangular faces on the cylinder are divided diagonally (essentially 2 triangles forming the rectangles), so it won't let me loop around it. :/ At least I think that's the problem. Is there any way to get rid of the divider lines so that I just have rectangular faces rather than 2 triangles that form a rectangle? $\endgroup$
    – Christopher Henderson
    Commented Jul 16, 2017 at 1:05
  • $\begingroup$ Alt-J Tris to Quads will help for the long faces, but if the hexagons are triangle fan then you might want to just delete the faces there until you get the division worked out. $\endgroup$
    – Craig D Jones
    Commented Jul 16, 2017 at 1:47
  • $\begingroup$ Please see the edit to my answer with the pic of the stages $\endgroup$
    – Craig D Jones
    Commented Jul 16, 2017 at 2:02

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .