How do I get rid of the extra faces created when extruding inwards?

Question

I'm using Blender 2.81a on MacOS.

I am modelling the 'rook' chess piece, and I want to give it the tower shape with cuts in it. When I select the faces and extrude them down, it makes a hollow shape with the edges, vertices and faces still intact. How do I get rid of these extra faces?

I came across the boolean modifier as a solution but is there any other tool/process that I am unaware of.

↑ My state

↑ Desired shape (tower with cuts)

Answer 1

Blender 2.90+

This feature is available from version 2.90 (https://builder.blender.org/download/)).

It's called Extrude Manifold and is located in the toolbox T, under the "Extrude" dropdown, or by pressing Alt+E.

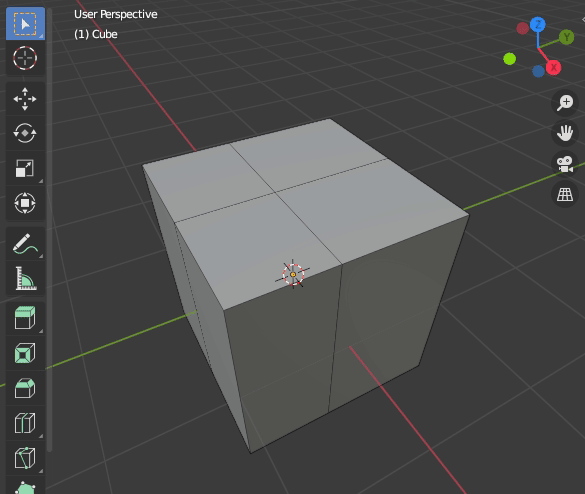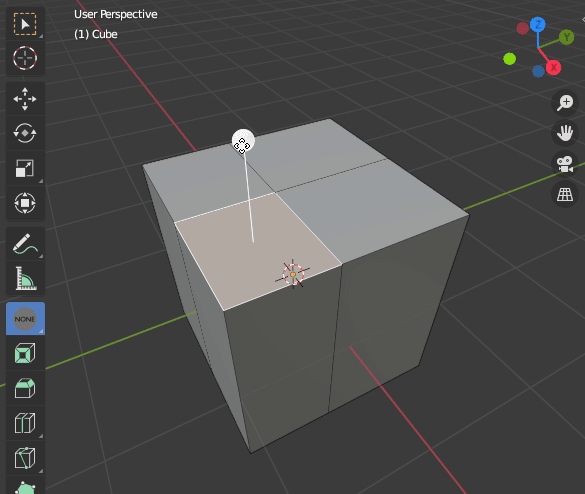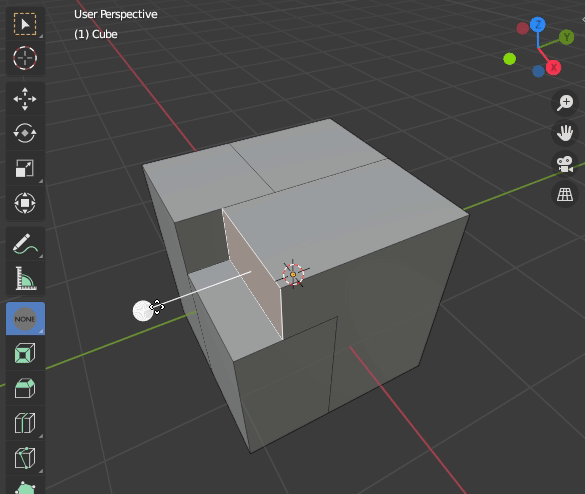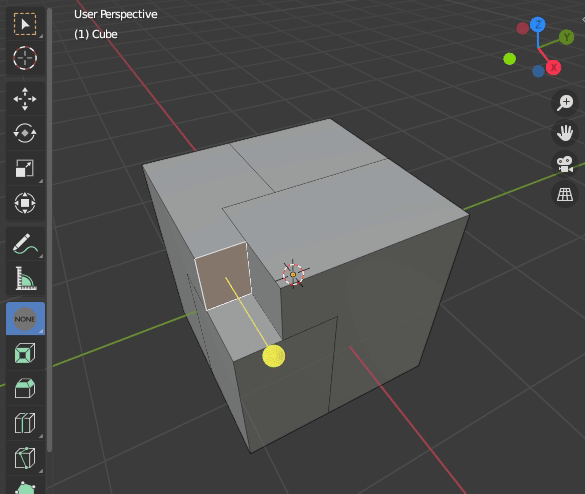
^{↑ Here I'm using Face select mode (3) but it works in vertex select too}

---

Blender Pre 2.90

You are expecting Extrude to work in the "Destructive" or "Push" mode,¹ i.e to be able to remove faces (and portions of them) as well as creating them. Unfortunately, Blender's Extrude doesn't currently offer that mode of operation.

For older versions of Blender, namely 2.79, there is a free user-contributed add-on that does that: Destructive Extrude for Blender, but it hasn't been updated for Blender 2.8x.

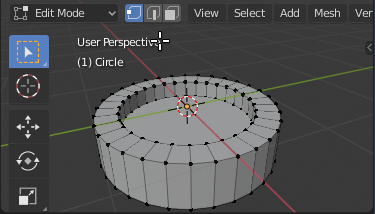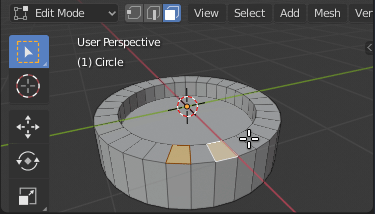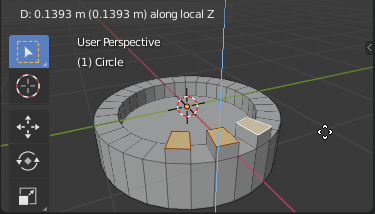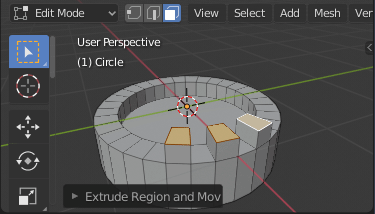
To model a mesh like the one you're after, you luckily have a very easy alternative: instead of extruding inwards, extrude outwards!

^{1. Using terminology from the Blender addon and from SketchUp respectively.}

Answer 2

If I was modeling it, I would have extruded those 'top brick-like' parts up instead of down and then scale the top part to the right size afterward. If you do want to extrude them downward, you would probably need to make a loop cut, delete some edges, and merge some vertices which is a bit more complicated solution to the problem.