3
$\begingroup$

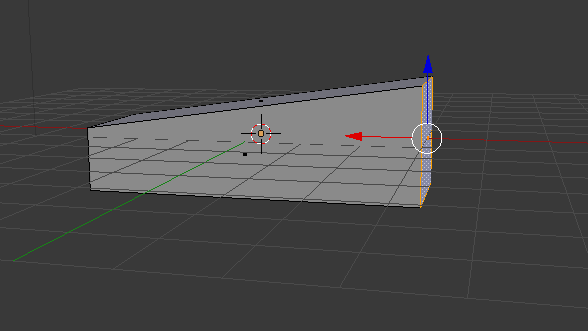
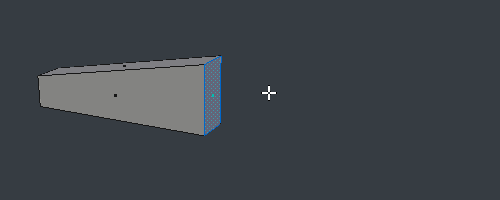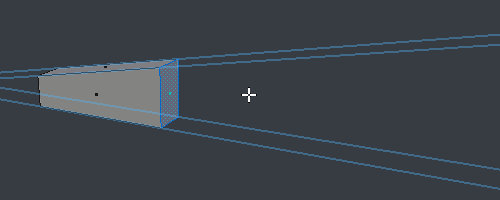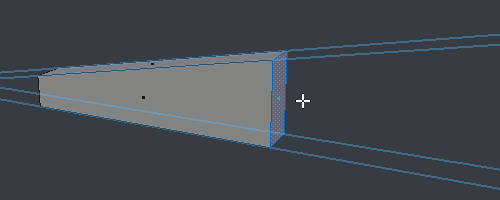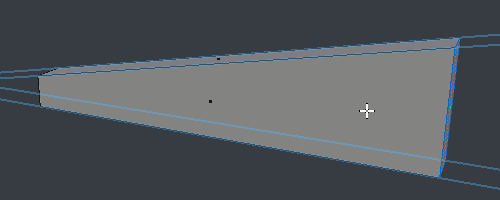
lets say i want to extend the object below just on his right side and it would not have the exact orientation at the x-axis like it has at the foto. so in other word: it should extend on the right side along his (long) edges (not at an axis). Is there a way to do that?

the edge slide does a similar thing i want but with that i just can make the object shorter but i want to have it longer on the right side.

PS: that object below is just an example not the one i am trying to work on

enter image description here

Improve this question
$\endgroup$
1
  • $\begingroup$ Press "e" to extrude, it should default to constrained axis. $\endgroup$
    – rob
    Commented Jan 3, 2019 at 14:49

1 Answer 1

Reset to default
5
$\begingroup$

You can still use edge slide to do this.

  • Select the end face (or its edges or vertices)
  • GG.. edge slide
  • Draw the face inwards a little way, making the piece shorter as you described in your post (this establishes the slide directions)...
  • Now hold down Alt, or tap C releasing the clamp. You should see rays extending the longitudinal edges.
  • As long as you are holding Alt, you can slide the end face's vertices anywhere you like along those rays.

enter image description here

Unfortunately, snap doesn't work with GG. If you wanted that, too, I think you would have to break the operation up, separately moving components along Custom Orientations derived from the longitudinal edges.

Improve this answer
$\endgroup$
3
  • $\begingroup$ thanks both for answers. the second one was exactly what i was looking for. $\endgroup$
    – Flaka
    Commented Jan 3, 2019 at 18:33
  • $\begingroup$ Is it possible to input a distance? $\endgroup$
    – batFINGER
    Commented Oct 31, 2020 at 13:16
  • $\begingroup$ @batFINGER In a a sense.. (you've got me looking).. If you're not set to 'Even' then the distance is expressed as a percentage of the length of the edges you're sliding along. Fair enough. If you are set to 'Even' sliding inwards, you can numerically enter an absolute length from the adjacent loop. However, I can't get that to work outwards, directly.. so you would have to do it in 2 steps: take your loop out too far, and then back, numerically, to the desired gap. $\endgroup$
    – Robin Betts ♦
    Commented Oct 31, 2020 at 14:07

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .