Curving a bevel? How to creature a unique radius on a T-junction?

Question

first time posting here since trying to figure this out on my own, and googling it has yet to give me a satisfactory answer.

I'm am trying to get more into hard surface modeling, so I thought I give myself a challenge, I probably bit off more then I chew at this moment.

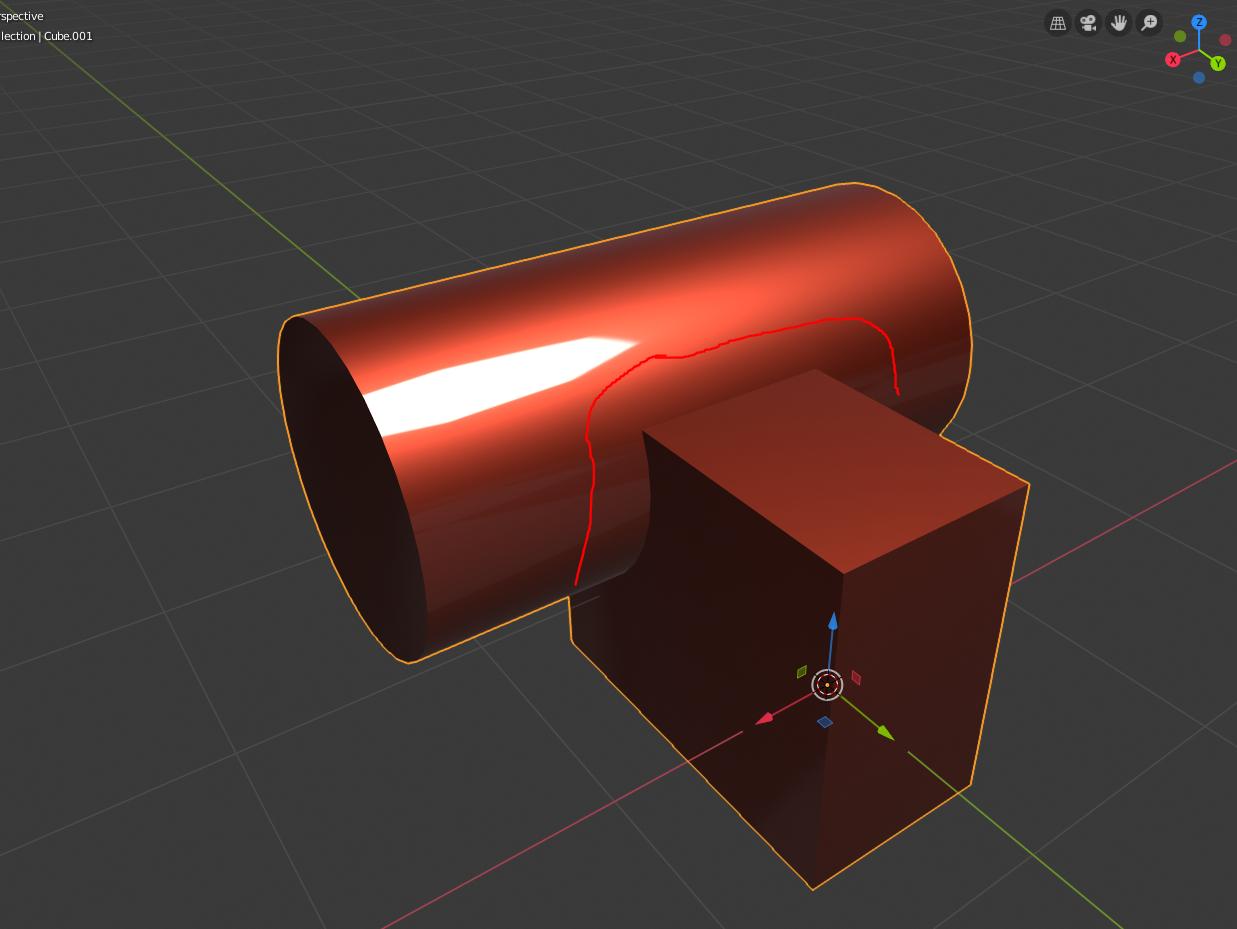
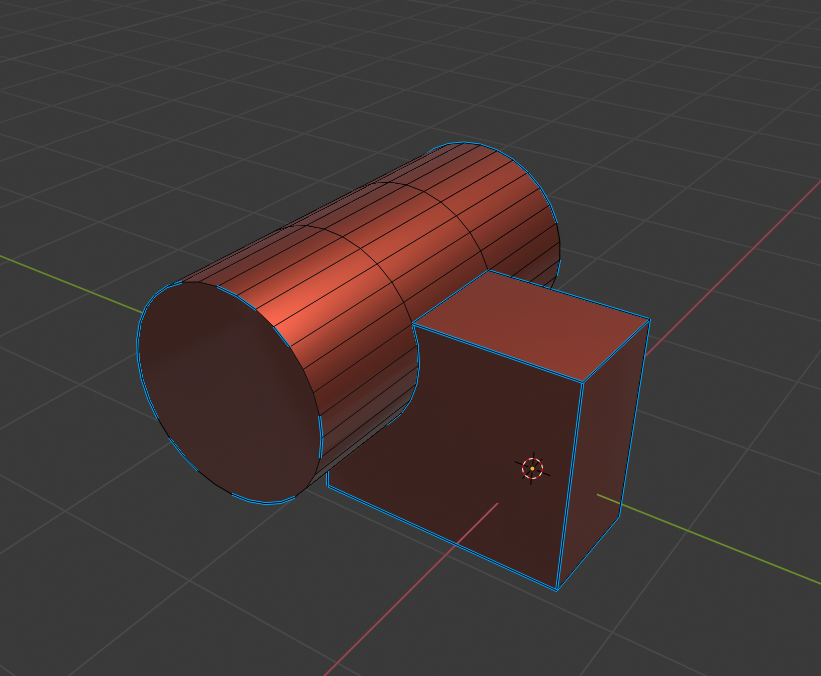
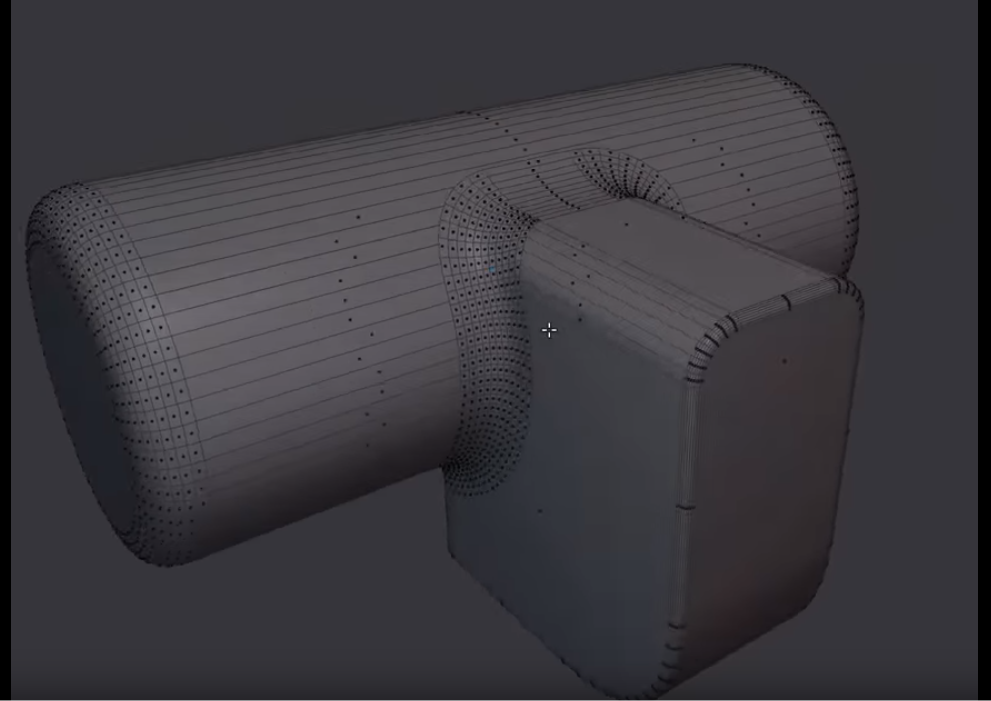
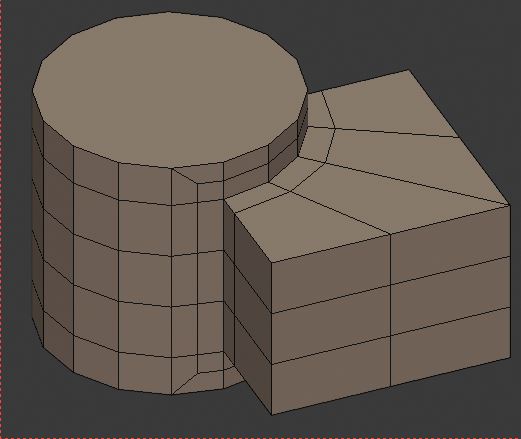
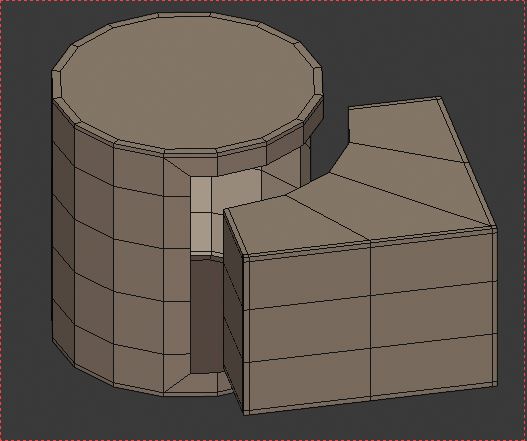
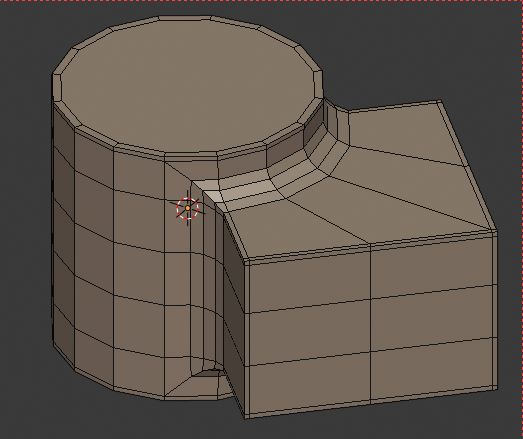
I am trying to create a bevel to fit around this unorthodox t-junction between a cylinder and a cube. I've tried regular beveling but it isn't quite giving me the results I want.

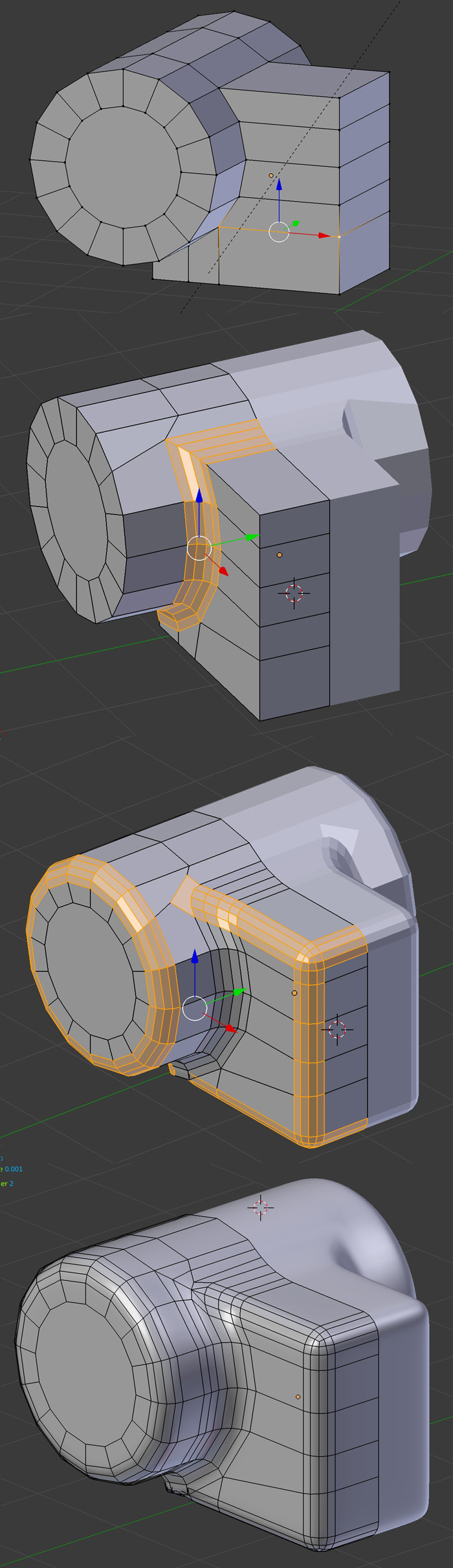
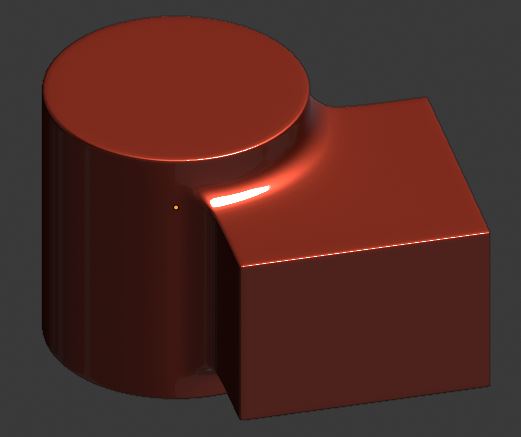
I'm more or less attempting to create a beautiful bevel similar if not exactly like this one in a video showcasing MESHMAchines Offset cut (The plugin I have purchased, it is really a brilliant plugin, still much to learn with its complexity)

So any recommendations on how I should approach this to figure out a solution to this puzzling bevel question?

Regards,

Answer 1

You could begin by a boolean, then work a bit with the knife to make sure that you only have quads, then bevel the welding, then the rest of the object, and bring some corrections to keep quads again:

Answer 2

An approach using Bridge Edge Loops.. this may or may not be the style of fillet you're looking for, but the tool gives a lot of flexibility.

.. arrange isolating face rings either side of the junction, looping all the way round on the cuboid, and 'tied off' at the corners on the cylinder.

.. delete the isolating rings, and bevel the convex edges of the cuboid, Segments 2, (this example has set Profile 1, and added holding edges at the top and bottom of the cylinder).

This restores the match of vertex-counts on both sides of the gap, as if the cuboid had been a straight extrusion from the cylinder, so you can..

.. Bridge Edge Loops, (Blend Surfaces), with a couple of cuts, adjusting Profile and Smoothness to the desired result..

.. and it will Catmull-Clark subdivide smoothly.