2
$\begingroup$

Here are the steps:

  1. Create a cube: SHIFT+A > Mesh > Cube
  2. Enter Edit mode: TAB
  3. Unselect All: A
  4. Go into edge select mode : CTRL+TAB > Edge

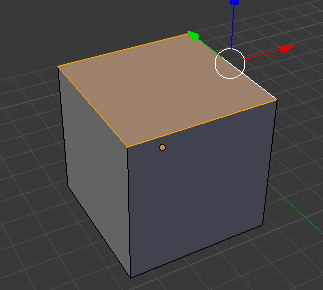
At this stage, just a side note: I will not be able to select a loop if I ALT+RMB on any edge... For my understanding, the 4 edges of the top face of the cube are a loop. In the below screenshot, I couldn't select those 4 edges with ALT+RMB.

I couldn't select those 4 edges with ALT+RMB

  1. Add a loop cut: CTRL+R
  2. Change to face selection: CTRL+TAB >Face
  3. Select All : A
  4. Delete all faces (only) : X > Only faces
  5. Change to edge selection: CTRL+TAB > Edge
  6. Try to select the previously inserted loop with ALT+RMB

=>It will not works... It will select all the edges

My question is: is it possible to select loop on object with no face?

Improve this question
$\endgroup$

1 Answer 1

Reset to default
1
$\begingroup$

For my understanding, the 4 edges of the top face of the cube are a loop.

Sure, they form a loop... but so do each of the other faces of the cube.

Each corner of the cube is a pole, where the edge flow can fork in either of three directions. So while it may be obvious to you which four edges you want to select, Blender has no way of knowing your intentions.

Improve this answer
$\endgroup$
3
  • $\begingroup$ But the question remains: Why isn't 'Loop selection' working if object only has edges? $\endgroup$
    – Baud
    Commented Dec 28, 2015 at 15:36
  • $\begingroup$ If the mesh "only has edges" and no faces, all connected edges will be selected when doing an edge loop selection. This is probably because to correctly assess where edge loops exist face normal data is required. But for the case in your example you are better off using Face Selection instead. $\endgroup$
    – Mentalist
    Commented Dec 29, 2015 at 2:38
  • $\begingroup$ Many thanks for this added input. If I understand well, there is no possibility to select a edge loop if the mesh has no face. I will wait one or two days to set this question as answered (in the case someone could come with a tip in the meantime). $\endgroup$
    – Baud
    Commented Dec 29, 2015 at 10:32

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .