1
$\begingroup$

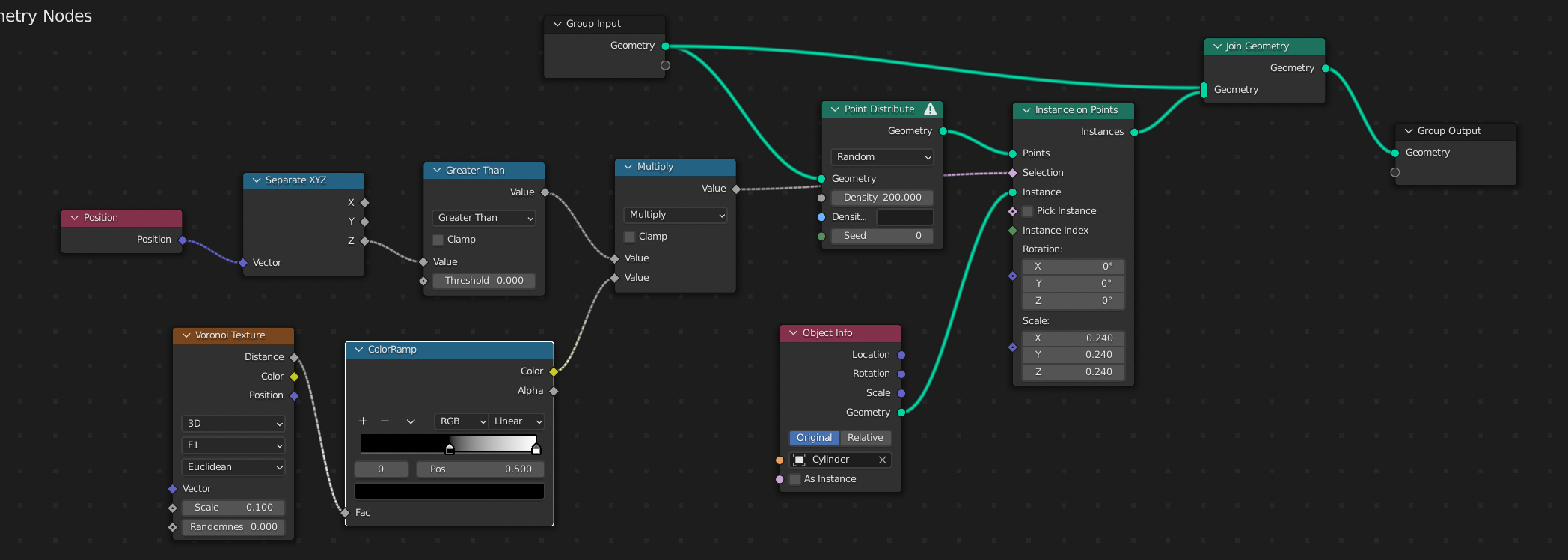
Problem statement

I have a simple node setup where I place some objects (grass collection) on a mesh if the z normal value is above 0.9. I would like this to follow a noise pattern (like the voronoi) such that it clusters together in some parts and to be completely absent in others.

I've tried using the Poisson disk option and control the density frac with the output of the noise node. This doesn't work and I don't understand why. The output from the noise is between 0 and 1 and the input to density is between 0 and 1. Is it taking the average instead of calculating it per point?

What are the alternatives for having multiple conditions for selecting points?

Thanks!

Edit

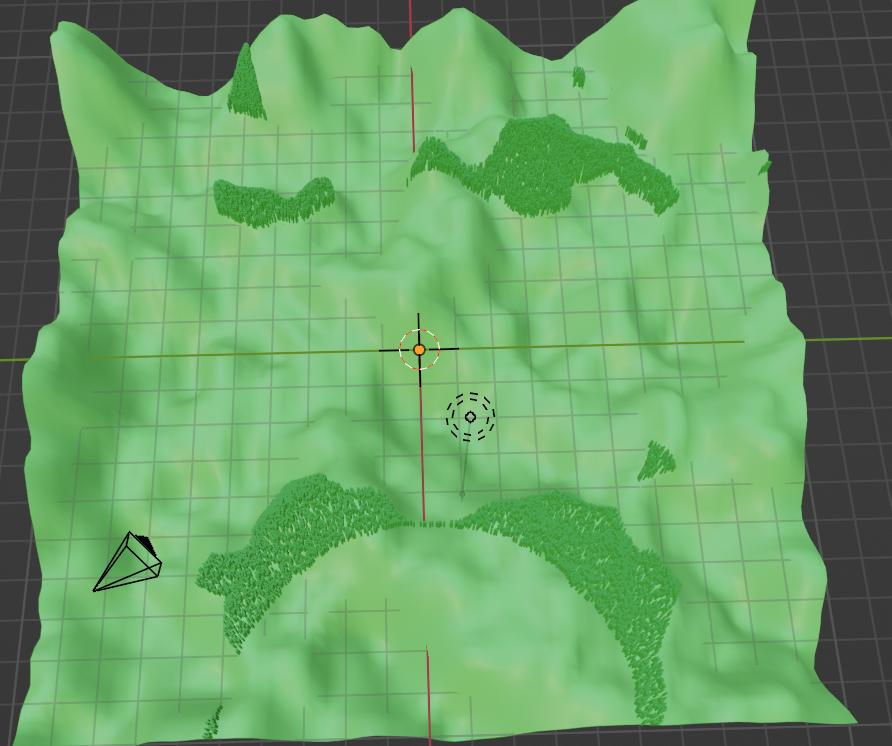
Thank you Chris for providing the exact setup I was looking for. I didn't think about using the color ramp.

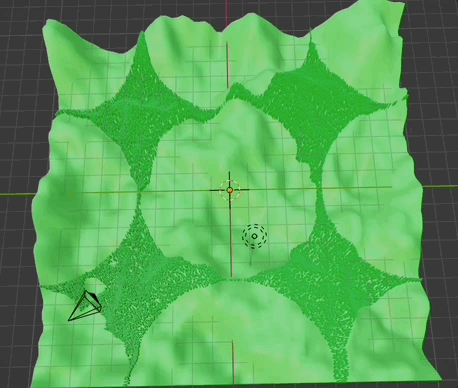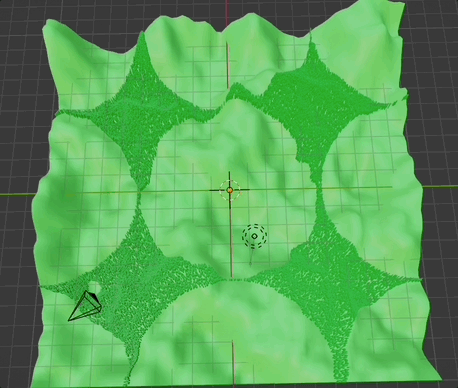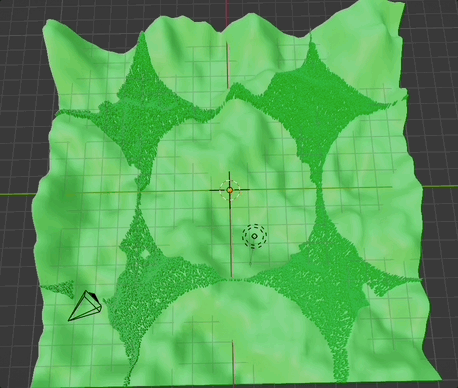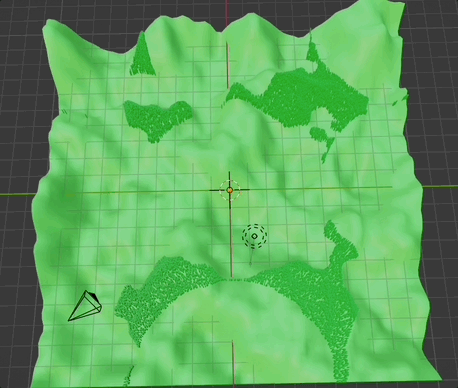
Also, Gorgious pointed out a very good problem! I was indeed only using a plane mesh and forgot about adding subdivisions.

Thank you very much for your help!

Improve this question
$\endgroup$
3
  • 1
    $\begingroup$ You base mesh needs to be subdivided enough because the density values are sampled at each vertex and interpolated on the polygon surfaces. Could you add a screenshot of your instancer mesh in wireframe mode ? $\endgroup$
    – Gorgious
    Commented Dec 23, 2021 at 10:07
  • $\begingroup$ please provide blend file (best) or at least your node tree so we can effectively help you... $\endgroup$
    – Chris
    Commented Dec 23, 2021 at 12:06
  • $\begingroup$ "normally" you would multiply if you have two logical and-conditions for your selection. Because selection just takes 0 (don't take into account) or 1 (take into account). For two logical or-conditions you can just take max(a,b) $\endgroup$
    – Chris
    Commented Dec 23, 2021 at 12:10

1 Answer 1

Reset to default
3
$\begingroup$

You can use this node setup (but i am only guessing what you want and what you want to achieve...)

enter image description here

result:

enter image description here

result when you change the threshold for z-value:

enter image description here

Improve this answer
$\endgroup$
1
  • $\begingroup$ Thank you! That's exactly what I was looking for! $\endgroup$
    – Anca Mihai
    Commented Dec 23, 2021 at 19:37

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .