2
$\begingroup$

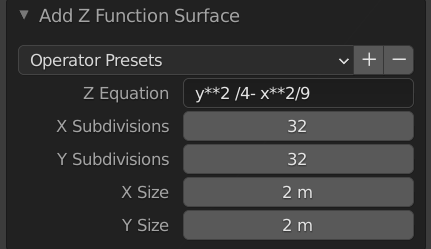
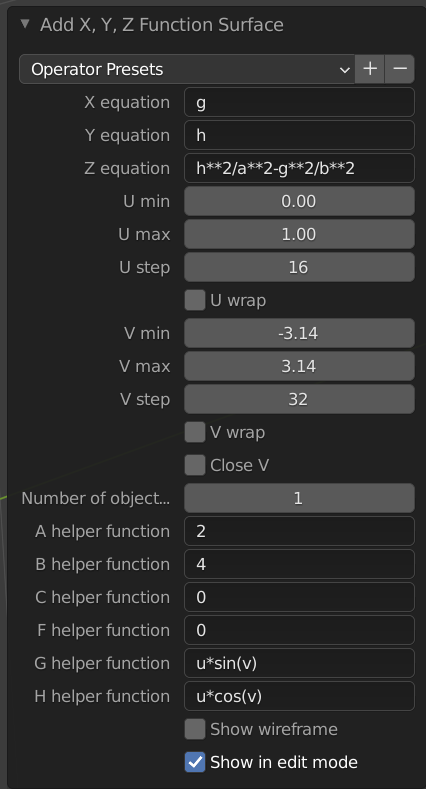
Would it be better done as a Z formula or an XYZ formula?

And if so, how?

According to this link:

http://mathandmultimedia.com/2011/04/15/potato-chips-and-mathematics/

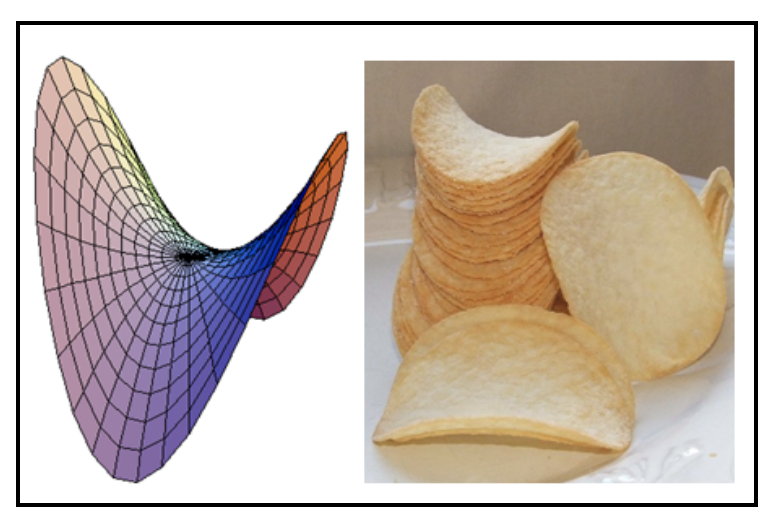
That would be, mathematically speaking a

"hyperbolic paraboloid quadratic and doubly ruled surface"

of cartesian equation as explained at the link above.

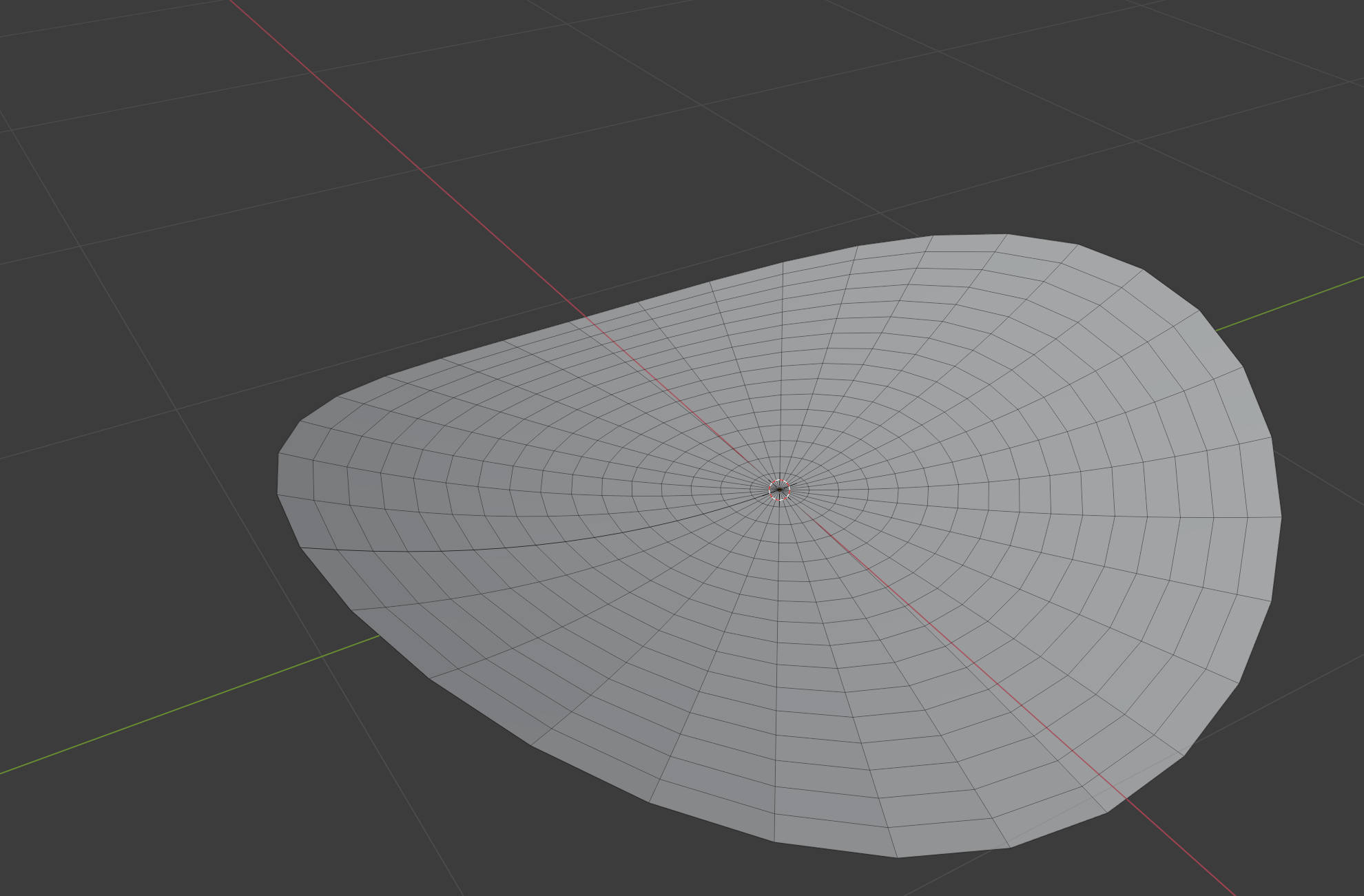
Not a hyperbolic paraboloid, saddle, as is commonly shown, but, rounded, all around

as shown below:

Pringle's Chip

Improve this question
$\endgroup$
6
  • 1
    $\begingroup$ Since you already know the analytic form as a Z function; it should definitely be a Z formula $\endgroup$
    – Marty Fouts
    Commented Aug 16, 2021 at 1:03
  • $\begingroup$ The way I read it, it translates to (yy / bb) - (xx / aa). Z formula has no a or b variables. Only x or y. So, how would I factor in a or b? Assigning a random integer as a constant for a or b in that formulation isn't working. And it's entirely possible that I'm off in left field. $\endgroup$
    – unkerjay
    Commented Aug 16, 2021 at 4:03
  • $\begingroup$ Ok, worked out the proper interpretation (y^2 / 2^2) - (x^2 / 2^2) [ Except without the ^ - the other way] - but that gives me an un-rounded hyperbolic paraboloid. Close. But no cigar. $\endgroup$
    – unkerjay
    Commented Aug 16, 2021 at 4:09
  • $\begingroup$ I've got this formula mod (XYZ - Enneper) from a previous question here. It looks close, but, it's not a Z formula: imgur.com/a/XZh422I $\endgroup$
    – unkerjay
    Commented Aug 16, 2021 at 4:46
  • $\begingroup$ StackExchange (Enneper) - Here: blender.stackexchange.com/questions/194257/… $\endgroup$
    – unkerjay
    Commented Aug 16, 2021 at 5:25

1 Answer 1

Reset to default
1
$\begingroup$

I offered the comment that you should use a Z formula but I neglected that a Z formula would give you a square potato chip.

surface y2/4-x2/9

That's a Z function that uses 2 as a and 3 as b.

To get a round potato chip you would use an XYZ surface:

the same as an XYZ function

Here is what it looks like

the potato chip

Explanation:

To get the round shape I used polar coordinates. I used u to represent the polar radius and v to represent the polar angle, in radians. The two helper functions g and h convert the polar coordinates for x and y. The values in the helper functions a and b control how 'bendy' the chip is along the respective axis.

Improve this answer
$\endgroup$
7
  • $\begingroup$ It's close Marty, but, if you look at the image, you've got it right on the sides, but it looks more like a pancake folded at the edges than the Pringles chip in the example image. As I look at it, it goes up on the left / right. It needs to come down on the front and back. $\endgroup$
    – unkerjay
    Commented Aug 16, 2021 at 20:50
  • $\begingroup$ It's a matter of tuning the settings, particularly a and b. I just picked two numbers at random. If I wanted to do a chip I'd add a subsurf after a solidify also $\endgroup$
    – Marty Fouts
    Commented Aug 16, 2021 at 20:54
  • $\begingroup$ I was just going to add, playing with a and b brought me closer to the mark: imgur.com/a/S9DQ2zj $\endgroup$
    – unkerjay
    Commented Aug 16, 2021 at 20:56
  • $\begingroup$ Noticed one small, common problem. as shown: imgur.com/a/S9DQ2zj I tried merge by distance, played around with the subd settings and added a displace. Still visible. I think it would go away with a wireframe though. $\endgroup$
    – unkerjay
    Commented Aug 16, 2021 at 21:07
  • $\begingroup$ Make sure that U wrap is off, that V wrap is on, and that Close V is off. If you don't wrap V then floating point math bites you. You'll also have to merge by distance $\endgroup$
    – Marty Fouts
    Commented Aug 16, 2021 at 21:12

Not the answer you're looking for? Browse other questions tagged .