2
$\begingroup$

Let's say that there is a sphere whose x,y,z dimensions are d. So, the circumference is d*pi. Now, the sphere is rolling on a surface. The z will stay the same, but the x,y locations are moved from (x1, y1) to (x2, y2). Assuming that the initial rotation is (0,0,0), can I calculate the rotation values for the last frame, so that I could make the rolling animation realistic? I mean, the sphere has some texture so that if it does not rotate, it will look as if it is sliding on the surface.

Improve this question
$\endgroup$

2 Answers 2

Reset to default
4
$\begingroup$

Simple driver

For constant rotation of a sphere / circle

enter image description here Think I used a poor choice of frame rate for gif

The distance a rolling circle travels is radius * angle with angle in radians. Circumference is special case of arc length. Gives the distance traveled for one full revolution, or 360 degrees or 2 * pi radians.

The angle theta rolled for a distance d

theta = d / radius

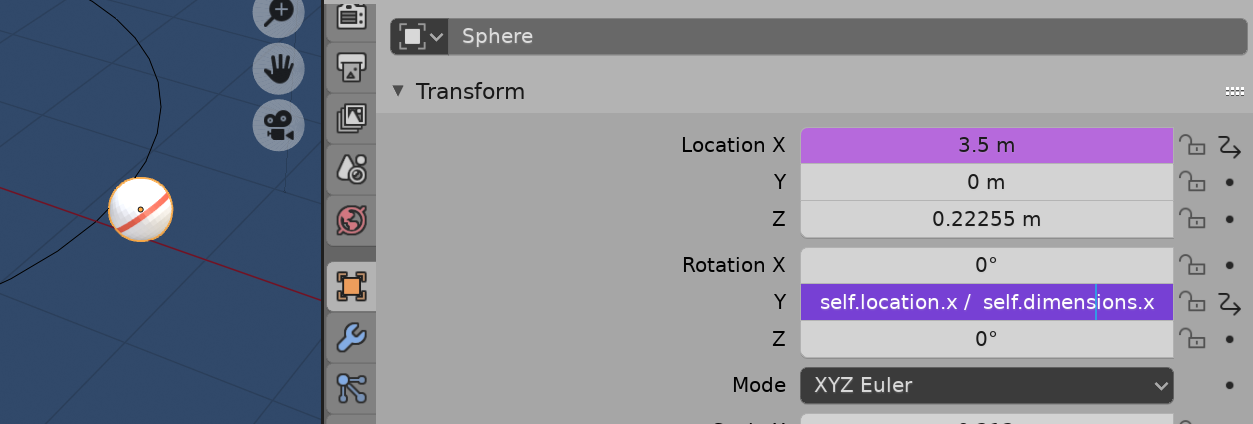
Set up a simple driver on the y rotation of a sphere based on its x location, and half a dimension as radius. Using self on drivers rather than a more traditional driver variable approach (Which removes warning)

enter image description here Drivers Editior

enter image description here Properties panel

2 * self.location.x /  self.dimensions.x

any dimension (diameter) could be used, or hardcoded

Using this our ball rolls about y as it travels along x.

To change the direction, possibly the simplest is to parent the whole setup to an empty. Or use angle axis rotation

Improve this answer
$\endgroup$
2
$\begingroup$

If you really want to do a realistic animation you should use rigid bodies. It's very easy. Using maths your gonna get crazy because there is a lot of things to consider: gravity friction etc there is a tutorial: https://www.youtube.com/watch?v=_WWmGp4jEog

Improve this answer
$\endgroup$
2
  • 1
    $\begingroup$ Thanks. I wasn't trying to be perfectly realistic, so gravity, friction, etc are probably not needed. And I assumed a flat surface. And moving a little bit (maybe about half of the circumference). I wondered what the last frame's rotation should be to make it look like rolling. Even in that case, is the calculation complicated? $\endgroup$
    – Damn Vegetables
    Commented Jan 25, 2020 at 6:03
  • 2
    $\begingroup$ If using rigids is dead easy, please help making this site better and add some steps on how to achieve this to your answer, links always can go down. Cheers! $\endgroup$
    – p2or
    Commented Jan 25, 2020 at 12:42

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .