I want to align the axis of a model to three points with a Python script.
How do I do this?
I pick three points that form a triangle with a right angle like this:
I made point A the origin (0,0,0). Then I think I should rotate the model around the point A so that AB and AC are aligned to the axis, but I'm not sure how I can do this. Should I get each vector's angle that separated x,y,z? Or is there any option in Blender to use for this?
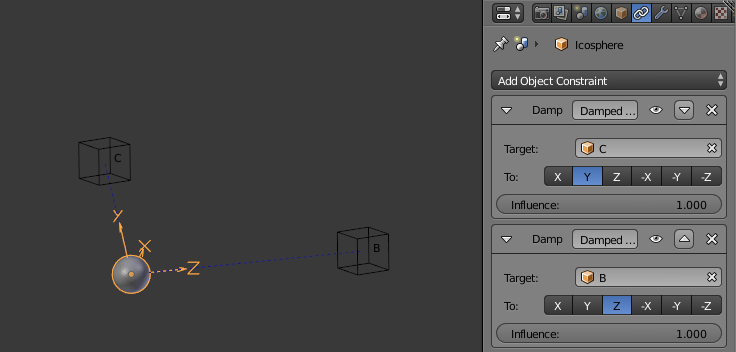
Create the matrix world
The rotation part of an objects world matrix is an orthogonal matrix where the columns represent the local axes.
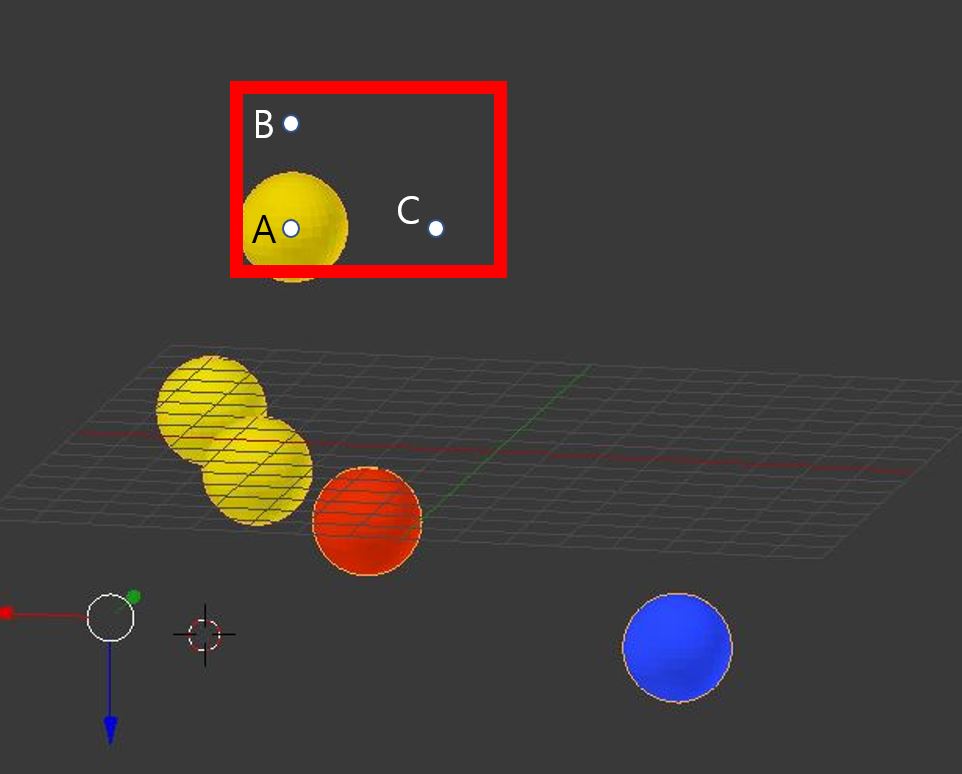
To demonstrate, in test code below, I have added a plane and removed one vertex to visualize the three points A, B and C ensuring the angle between BA and CA is 90 degrees.
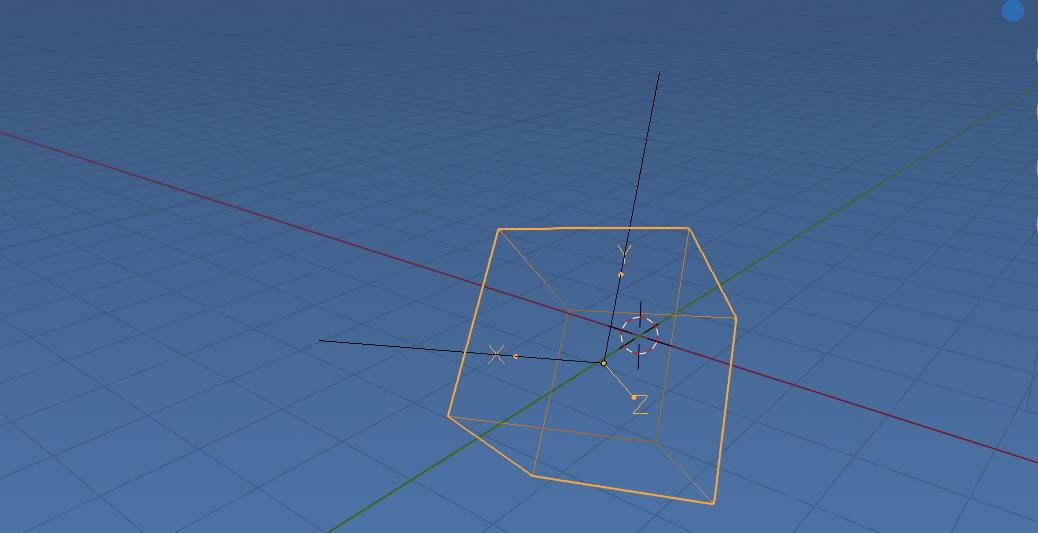Cube (orange wireframe) aligned to 3 vertex, 2 edge "plane" Vertex 0 for B, 1 for A and 2 for C
The vectors BA and CA, normalized,, are used for the x and y axis directions.
The cross product of x and y is the z axis. (The negated value would be the "flipped" option)
Finally build the matrix world from the 3 axis vectors, make it 4x4 and the translation A.
Test script. Move and rotate the "plane" then run script to align "Cube" such that its origin is at A, and the x and y axis align.
import bpy
from mathutils import Matrix
context = bpy.context
scene = context.scene
tri = scene.objects.get("Plane")
# global coords of 3 verts in plane.
b, a, c = [tri.matrix_world @ v.co for v in tri.data.vertices]
x = (b - a).normalized()
y = (c - a).normalized()
z = x.cross(y)
M = Matrix([x, y, z]).transposed().to_4x4()
M.translation = a
# Cube is the test object
ob = scene.objects.get("Cube")
ob.matrix_world = M
Note I have used blender 2.8. Replace matrix - vector multiplication @ with * for prior versions.
You can use Damped Track constraints.
