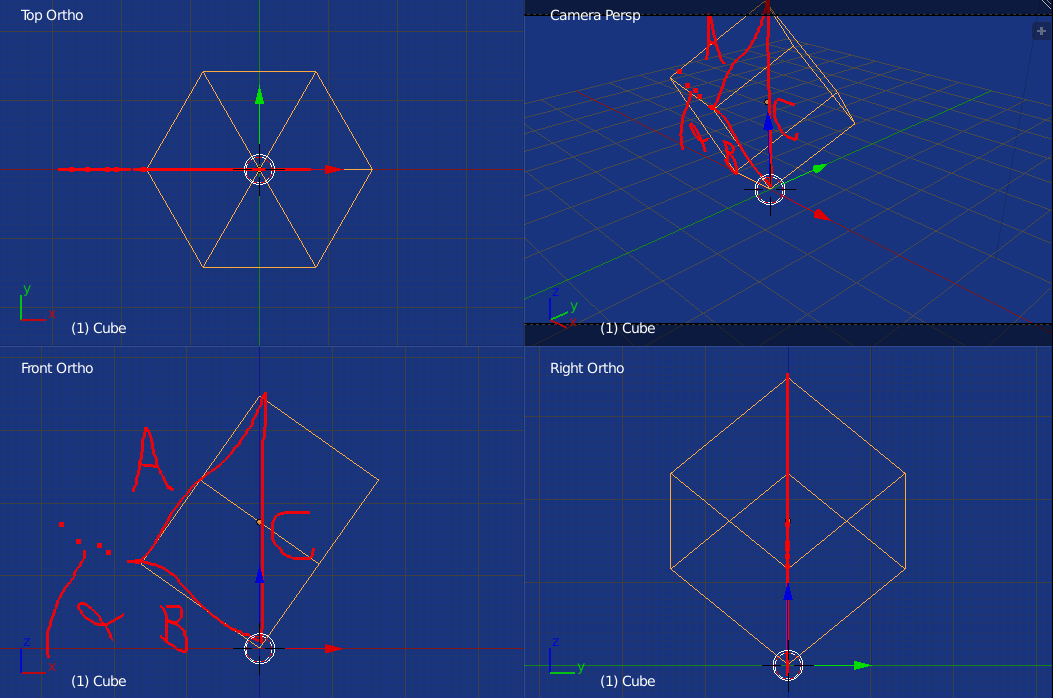
Mathematically
if you look at the Front Ortho of the image there is the triangle ABC. Length B is the side of the cube L, length A is the length of a faces diagonal L * sqrt(2) L * sqrt(2) (basic pythagoras). The tangent of angle alpha will be L / (L * sqrt(2))L / (L * sqrt(2)) or 1 / sqrt(2) atan1 / sqrt(2) atan of which equates to 35.2644 degrees.
The rotation_euler XYZ (45, 35.264, 0)(45, 35.264, 0)
The length of the longest diagonal C is L * sqrt(3)L * sqrt(3), Once again from pythagoras. If the origin is in the middle of the cube translate the cube L * sqrt(3) / 2L * sqrt(3) / 2 to put it on its point.
Interestinglythe volume can be calculated using C**3 / (3*sqrt(3))C**3 / (3*sqrt(3))