I read "How do I create repeating patterns in cycles with procedural textures?" here on Blender Stack Exchange, and "staggered texture mapping" on Greg Zaal's website. I thought it seemed possible to combine the two ideas to have staggered procedural tiles, but can't seem to make it work. Can anyone please help?
2 Answers
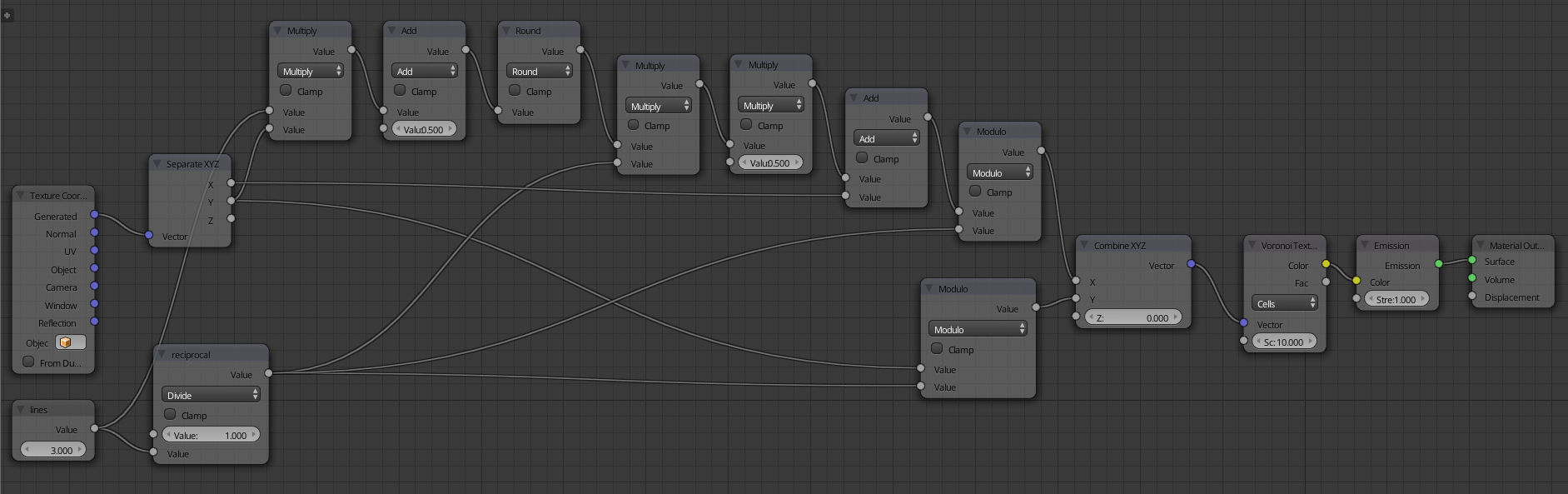
Here are the nodes for creating staggered texture mapping for procedural textures.
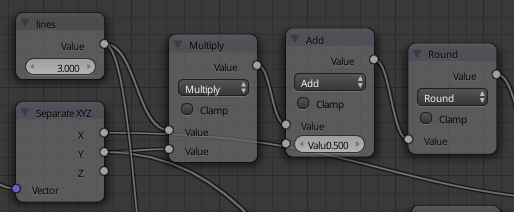
 First the Y axis gets multiplied by the number of lines you want to have in the pattern (the Lines value node). After adding
First the Y axis gets multiplied by the number of lines you want to have in the pattern (the Lines value node). After adding .5 that gets rounded creating stripes (along the X axis in this example).
- If you were to look at the output from that node at this point it would look all white, that is because the values start at 1 and increase by 1 with each stripe.
The next three nodes take the stripes, multiplies it by the reciprocal of the input value (lines), then by 0.5 to lower the values. Then the X axis is added in.
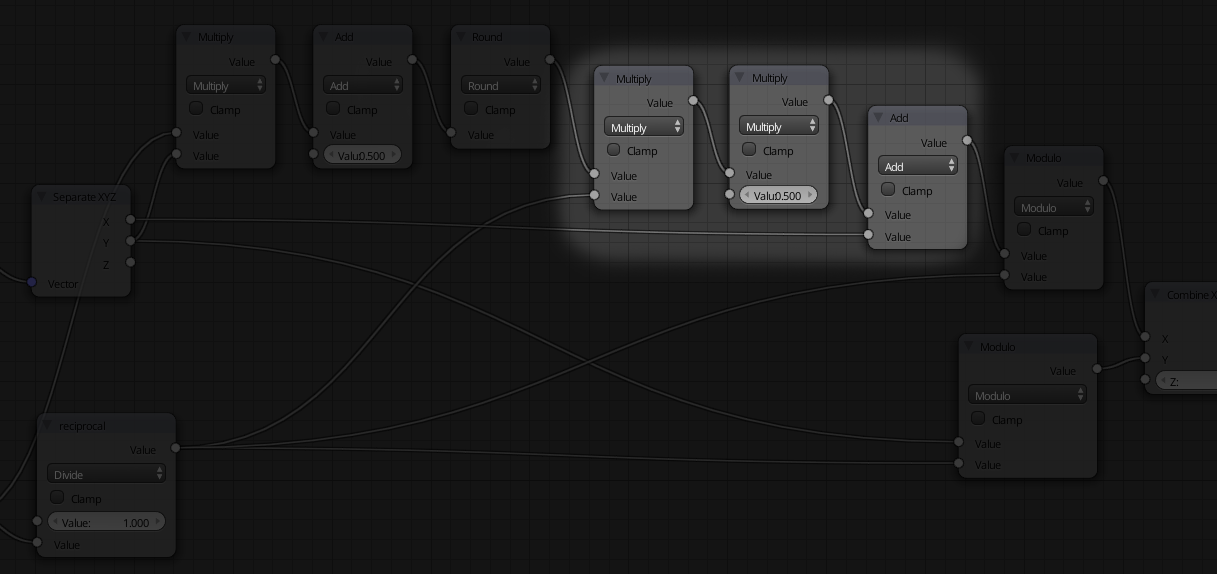
At this point there are only stripes, next the Modulo math node causes the texture coordinates to repeat.
All that was for the X axis.
The Y axis is simple, it is just the Modulo of the reciprocal of the lines.
With the nodes pictured at the top you can get a pattern like this.

After adding the Subtract and Absolute nodes from Jerryno's answer, every other tile will be mirrored and you can get a pattern like this.
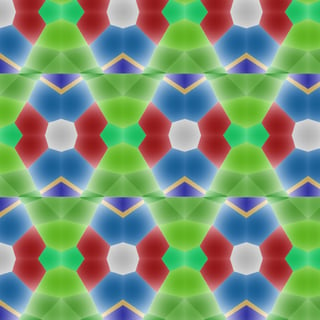
This blend has the nodes packed in to a node group, which can easily be added to any project.
Blend File
As you can see, manipulating the texture coordinates of generated textures is quite powerful.
-
1$\begingroup$ You are the king. This is exactly what I was looking for. Thank you!!!! $\endgroup$ Commented Apr 24, 2015 at 4:26
Try this one, but only on 2.8
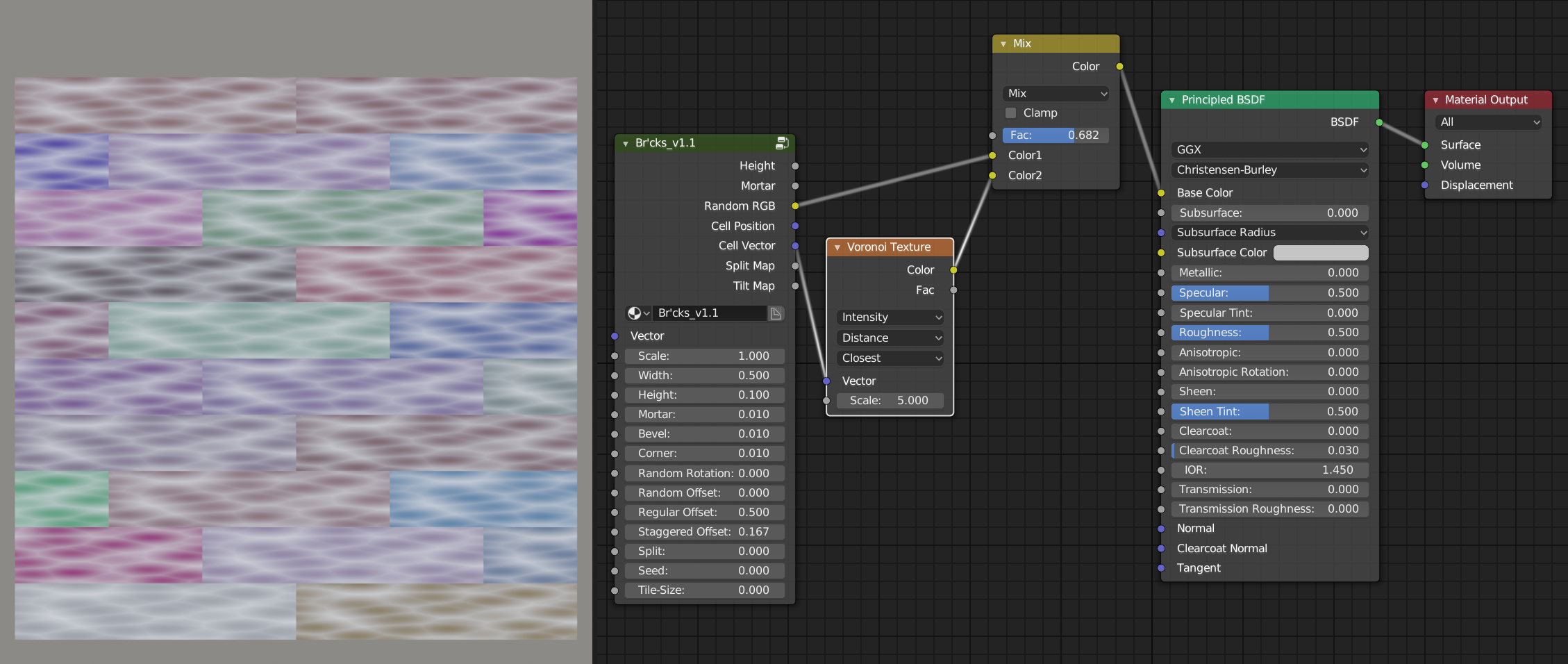
you can append "br`ck" node tree then use br`ck_v1.1 node like this
-
4$\begingroup$ While this link may answer the question, it is better to include the essential parts of the answer here and provide the link for reference. Link-only answers can become invalid if the linked page changes. - From Review $\endgroup$ Commented Apr 4, 2019 at 16:00
-
$\begingroup$ @Georges, thanks for correction. $\endgroup$ Commented Apr 5, 2019 at 3:19