2
$\begingroup$

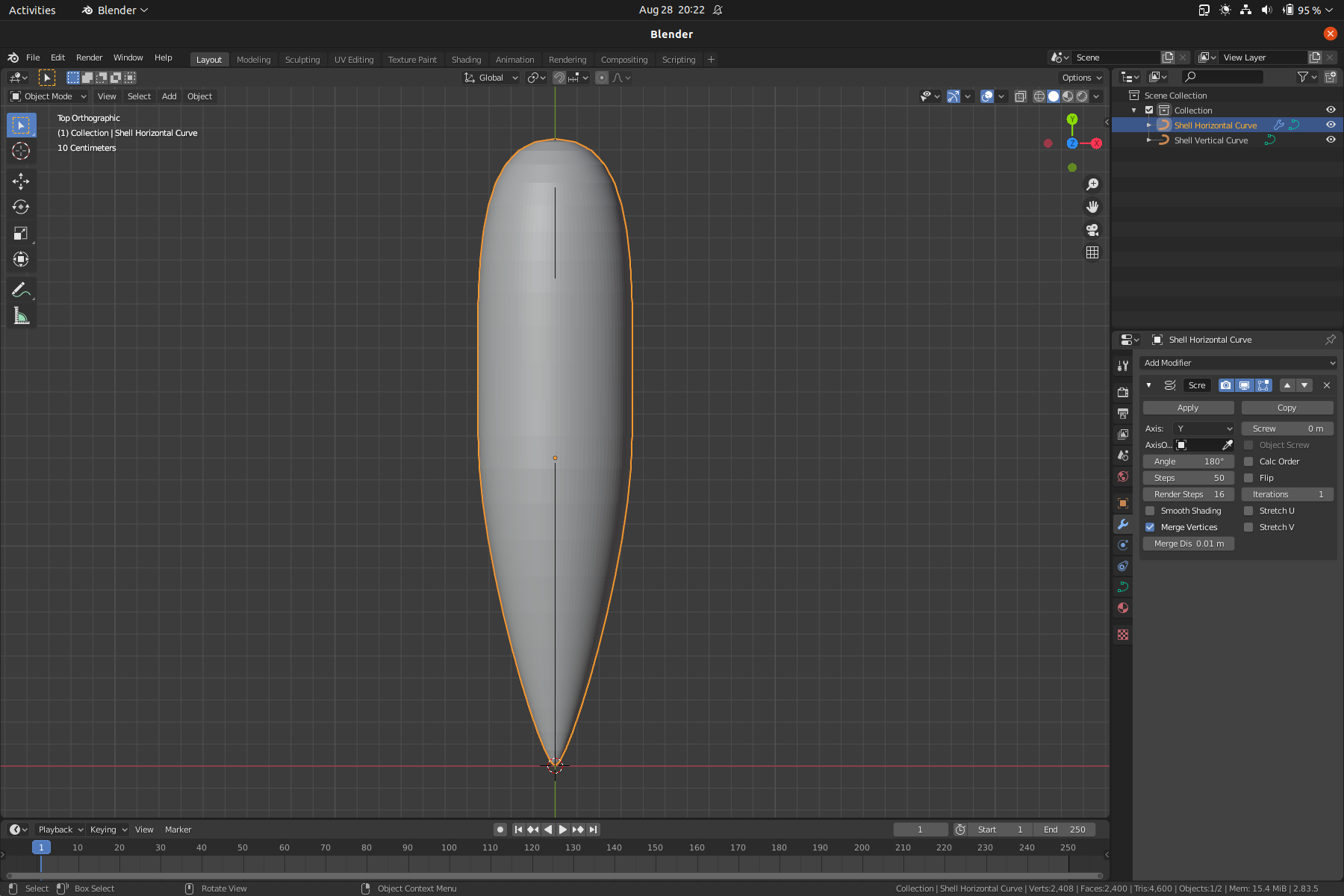
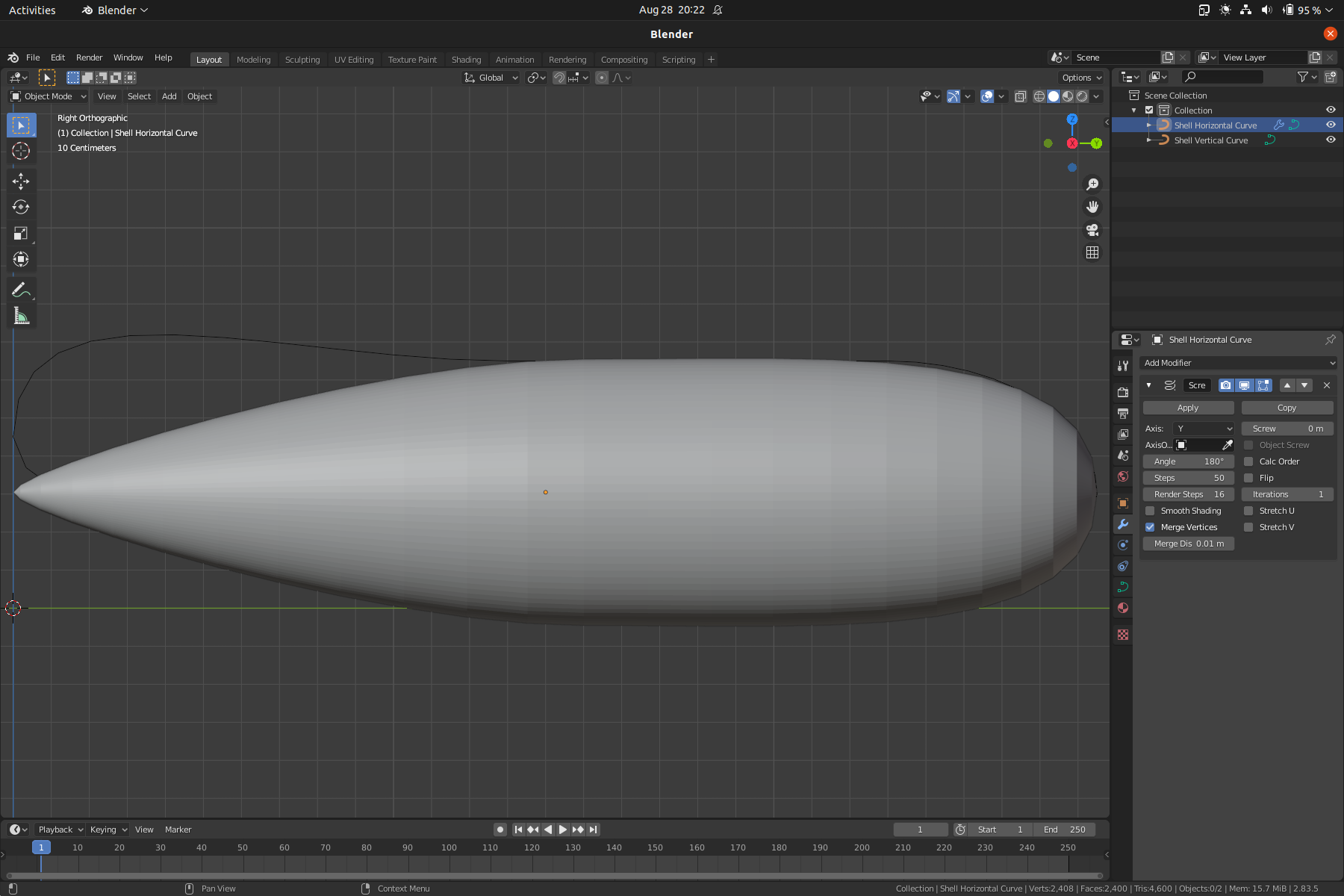
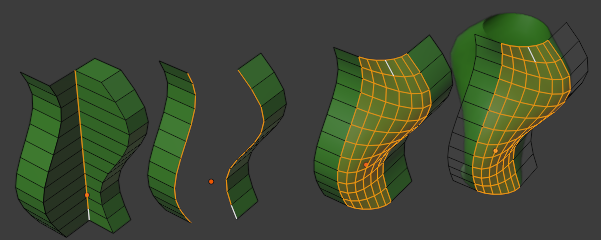
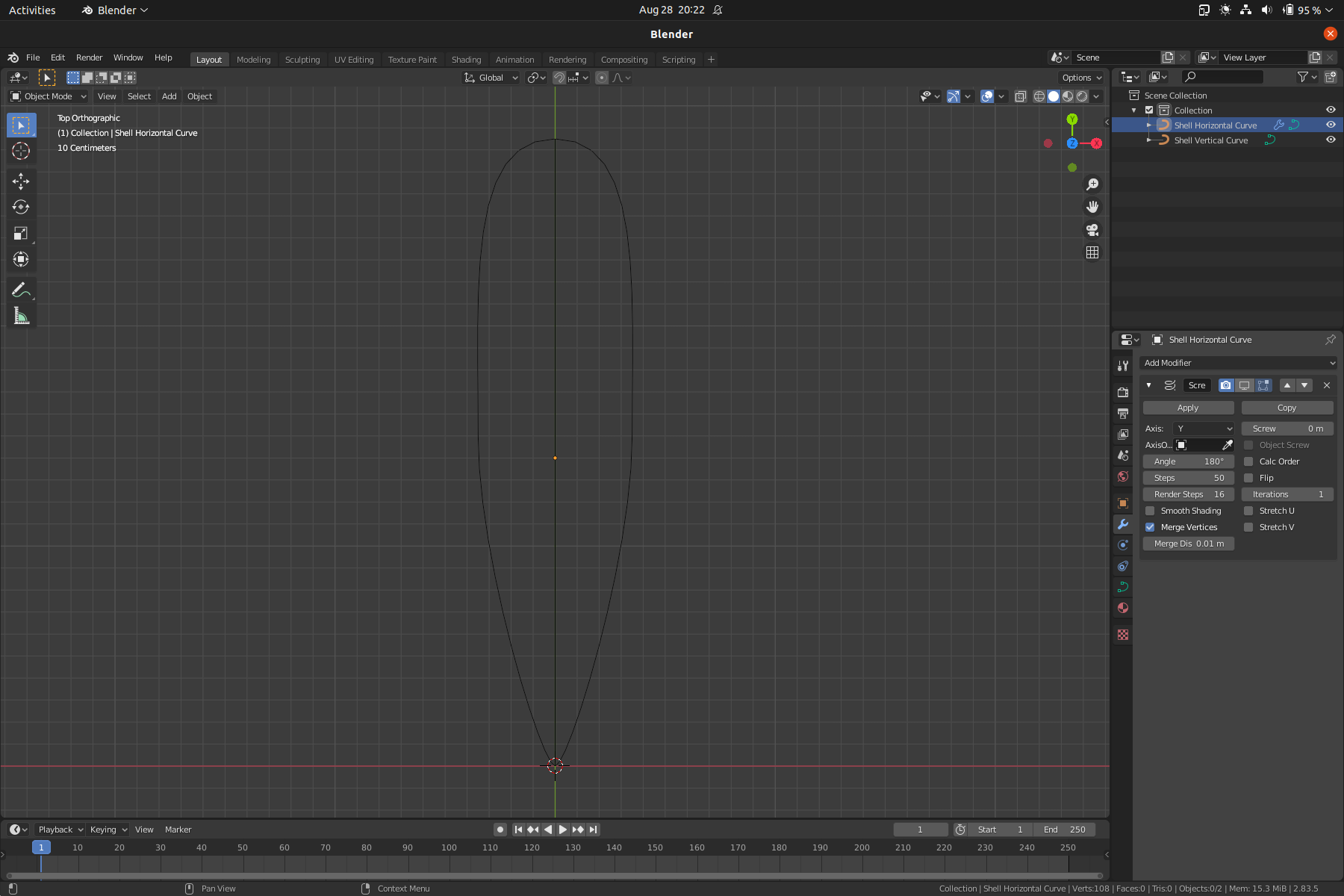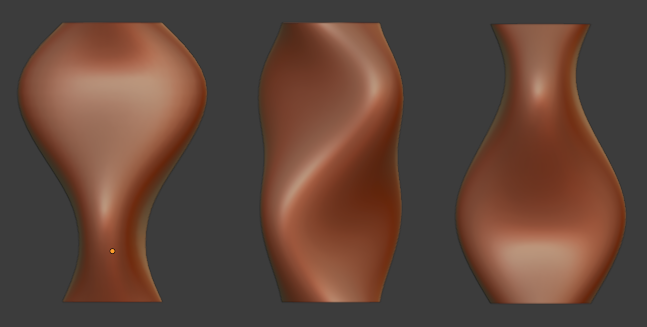I'm quite familiar with taking one curve and creating a mesh by revolving the curve around an axis, such as through the screw modifier. This creates symmetrical cross sections . However, what if the cross sections I want are not symmetrical? How can I create a solid of revolution that follows the shape of two different, perpendicular curves? Examples below:

Top-down view. This is the lateral shape I want:

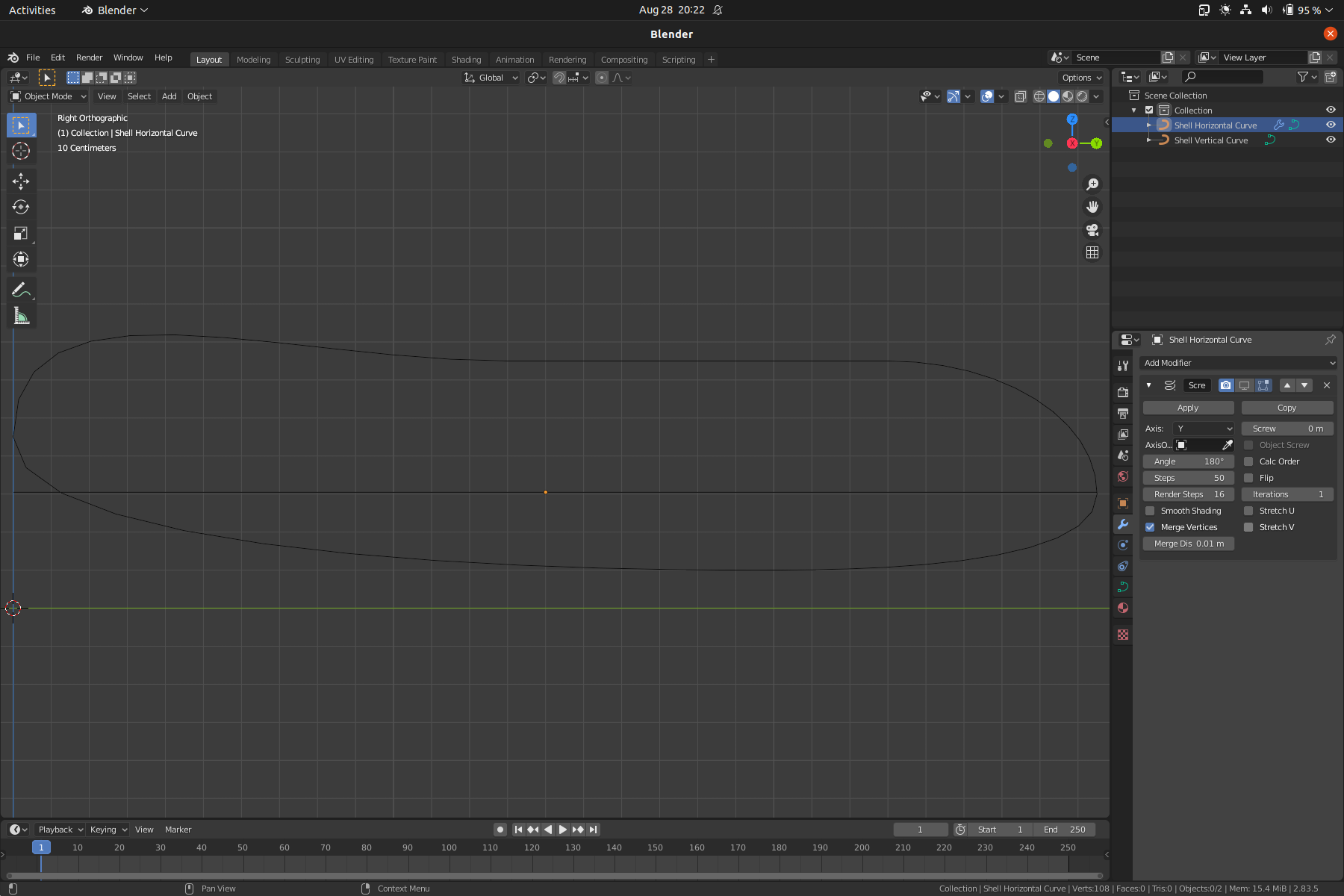
enter image description here

Without modifier: enter image description here

Side view. I want the first curve and revolution to conform to the second curve

enter image description here

Without modifier: enter image description here

So now, do I do some sort of deform, or something else? I thought the curve modifier might work, but my attempts resulted in something that looks like it belongs in a modern art museum. Any other techniques that might work better?

EDIT: Blend file linked below:

Improve this question
$\endgroup$

1 Answer 1

Reset to default
4
$\begingroup$

This isn't procedural, but ...

If the object has 2 profiles, it's not really a surface of revolution? It's an interpolation?

enter image description here

  • Here, the 2 profiles are in planes, to make them easier to see, at right-angles to one another, intersecting at the object origin, and extruded tangentially to guide 'Blend Surface',later.

  • Once done, the intersection of the planes is deleted

  • And the profiles are joined with Bridge Edge Loops, set to 'Blend Surface', with a few cuts.

  • Then the ¼ object has been Mirrored in 2 axes, with 'Bisect' set to get rid of the guiding extrusions.

enter image description here

Improve this answer
$\endgroup$
6
  • $\begingroup$ I'm definitely not getting intended results. Perhaps I haven't understood the instructions correctly. Or could it be that the curves have dissimilar vertices? $\endgroup$
    – hiigaran
    Commented Aug 28, 2021 at 12:25
  • $\begingroup$ @hiigaran For sure, there must be the same number of vertices in the profiles. Play with the settings in the Bridge Edge Loops F9 panel, too, to get used to them. $\endgroup$
    – Robin Betts ♦
    Commented Aug 28, 2021 at 12:31
  • $\begingroup$ Will do, thanks. Worst case scenario, I'll just make 0.1m intervals of circle curves as cross sections, then adjust as required. Not the most efficient solution if it does come to that, but at least there's a backup $\endgroup$
    – hiigaran
    Commented Aug 28, 2021 at 12:36
  • $\begingroup$ @hiigaran I've just had a look at your file.. one of the loops is asymmetrical, so that's 3 profiles. Is that what you want? $\endgroup$
    – Robin Betts ♦
    Commented Aug 28, 2021 at 12:55
  • $\begingroup$ The entire thing should be symmetrical on the Y axis, but that's it $\endgroup$
    – hiigaran
    Commented Aug 28, 2021 at 13:24

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .