Is it wise to use edge loops and subdivision modifiers on high poly meshes?

Question

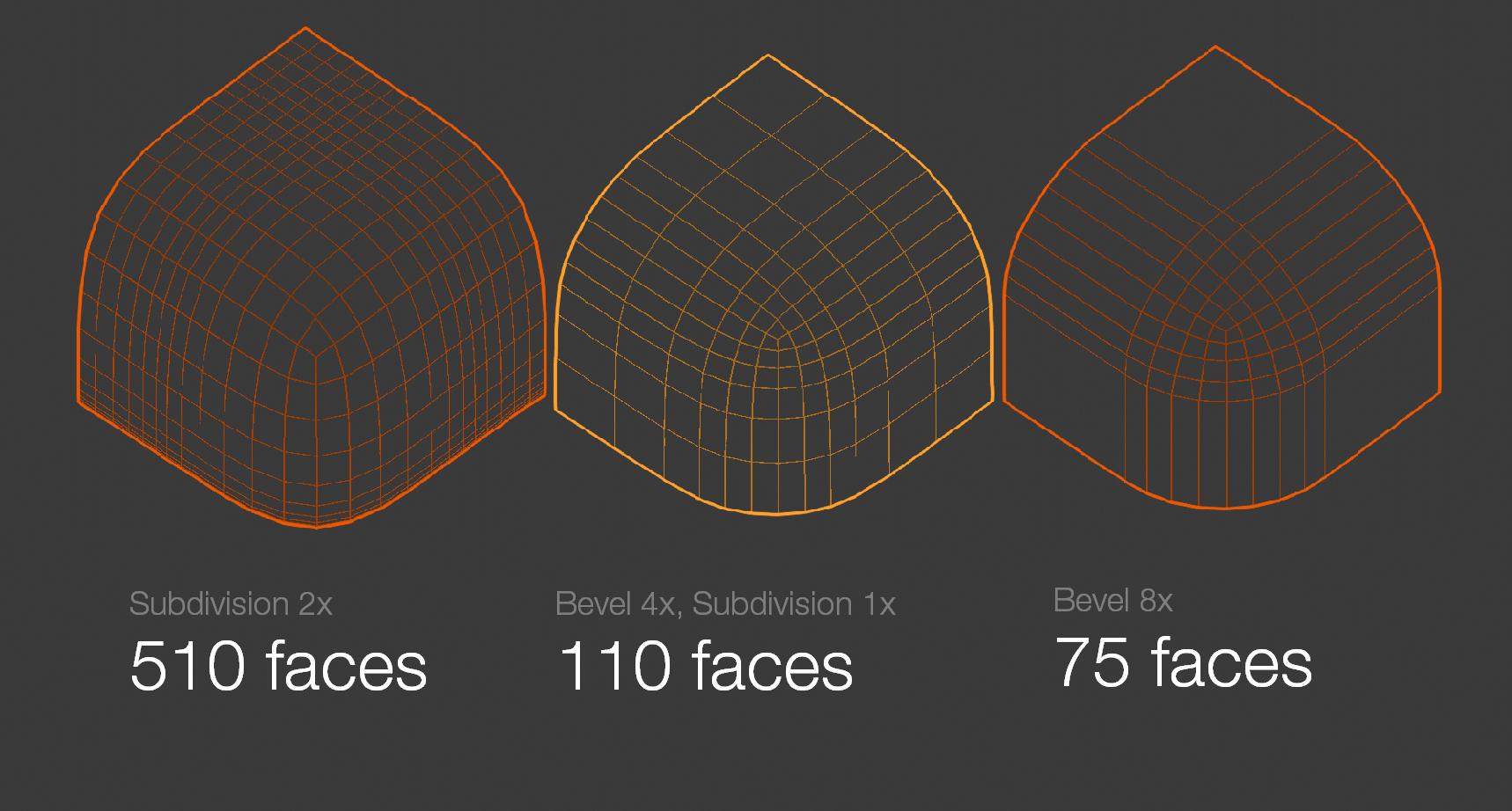
in Blender tutorials i see that apart from CTRL + E, loop cuts are often used to preserve edges, despite subdivision surface modifier.

Now that I have some experience with Blender and have created meshes with more than 100,000 faces, I wonder if this is the most useful method.

Of course it is faster to add a layer of the subdivision modifier and then add loopcuts, but the bottom line is that Blender has to work with more vertices and faces.

I am aware that if the mesh is not near the camera, you can set the subdivision modifier to adaptive (cycles) or use a decimate modifier.

Now to the real question: Is it better for performance to use a subdivision modifier lv 1 and add the details yourself to reduce vertices in the end result, or to work with loop-cuts and subdivision modifier lv 2 to reduce vertices in the viewport?

Answer 1

Lower polycount is better performance-wise.
But sometimes it makes sense to sacrifice performance for ease of use.

---

Bevel modifier
- easily adjustable edge width, great for hard surface modeling
- not suitable for complex corners and edges (creates overlaps)
- low-poly results

Subdivision Modifier
- easy to use, great for organic surfaces
- works almost anywhere
- hi-poly results (4x more polygons with each level)

In reality they're often used together.

Let's compare
Same edge resolution, different face count.